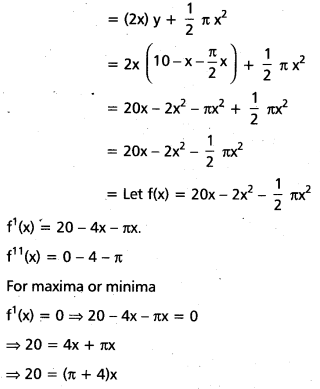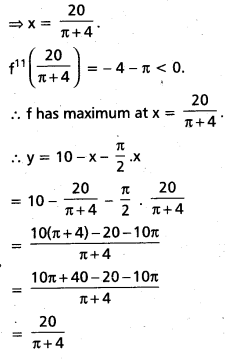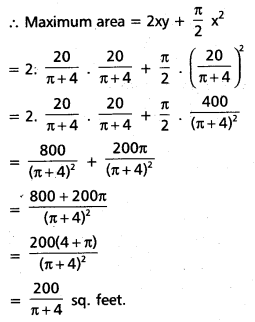TS Inter 1st Year Maths 1B Question Paper May 2019

Access to a variety of TS Inter 1st Year Maths 1B Model Papers and TS Inter 1st Year Maths 1B Question Paper May 2019 allows students to familiarize themselves with different question patterns.

TS Inter 1st Year Maths 1B Question Paper May 2019

Time : 3 Hours
Max. Marks : 75

Note : This question paper consists of THREE sections A, B and C.

Section – A
(10 × 2 = 20 Marks)

I. Very short answer type questions :

1. Answer all the questions.
2. Each question carries two marks.

Question 1.
Find the equation of the straight line which makes an angle 60° with the positive x-axis measured counter-clockwise and passing through the point (1, 2).
Solution:
Slope of the line m = tan 60° = \(\sqrt{3}\).
Required straight line equation is y-2=V3(x-1)
⇒ y – 2 = \(\sqrt{3}\)(x – 1),
⇒ y – 2 = \(\sqrt{3}\)x – \(\sqrt{3}\)
⇒ \(\sqrt{3}\)x – y + (2 – \(\sqrt{3}\)) = 0

Question 2.
Find the value of P, if the straight lines 3x + 7y – 1 = 0 and 7x – py + 3 = 0 are mutually perpendicular.
Solution:
Given straight line equations are
3x + 7y – 1 = 0 ……… (1)
7x – py + 3 = 0 ……. (2)
Since (1), (2) are mutually perpendicular.
∴ 3(7) + 7(-P) = 0
⇒ 21 – 7P = 0
⇒ 7P = 21
⇒ P = 3

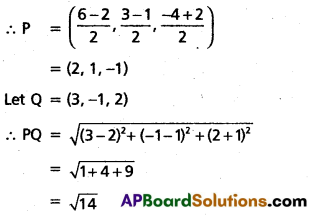
Question 3.
Find the distance between the mid point of the line segment \(\overline{\mathrm{AB}}\) and the point (3, -1, 2), where A = (6, 3, -4) and B = (-2, -1, 2).
Solution:
Given A = (6, 3, -4)
B = (-2, -1, 2)
Let P be the midpoint of the line segment \(\overline{\mathrm{AB}}\)

Question 4.
Find the angle between the planes x + 2y + 2z – 5 = 0 and 3x + 3y + 2z – 8 = 0.
Solution:
Given plane equations are
x + 2y + 2z – 5 = 0 ….. (1)
3x + 3y + 2z – 8 = 0 …… (2)
Let ‘θ’ be the angle between the planes (1) and (2)

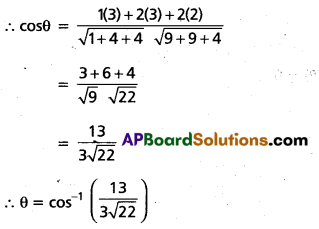
Question 5.
Find the left hand limit and right hand limit of the function, f(x) = \(\frac{|x-2|}{x-2}\) at x = 2.
Solution:
Given f(x) = \(\frac{|x-2|}{x-2}\), x ≠ 2

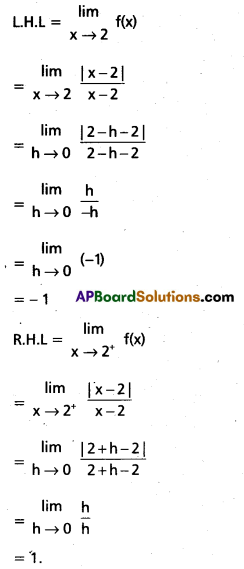
Question 6.
Compute :

Solution:

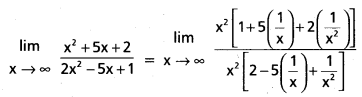
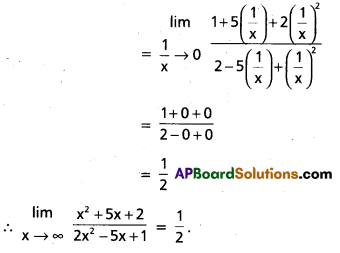
Question 7.
Find the derivative of the function, f(x) = 5 sinx + e^x Log x.
Solution:
Given f(x) = 5 sinx + e^x logx.
Differentiating w.r.to. ‘x’ on both sides, we have
f'(x) = 5cosx + e^x. \(\frac{1}{x}\) + logx.e^x
= 5 cosx + e^x(\(\frac{1}{x}\) + logx).

Question 8.
If x = e^{sin hy}, find \(\frac{\mathrm{dy}}{\mathrm{dx}}\).
Solution:
Given x = e^{sin hy}
Taking logarithm on both sides, we have
logx = sin hy
Differentiating w.r.to ‘x’ on both sides, we have
\(\frac{1}{x}\) = cos hy . \(\frac{d y}{d x}\)
⇒ \(\frac{d y}{d x}\) = \(\frac{1}{x \cosh y}\)

Question 9.
Find dy and ∆y of y = f(x) = x² + x at x = 10. when ∆x = 0.1.
Solution:
Given f(x) = x² + x, x = 10 and ∆x = 0.1
dy = f’(x) ∆x
= (2x + 1) ∆x
= [2(10) + 1] (0.1)
= (21) (0.1)
= 2.1
∆y = f(x + ∆x) – f(x)
= (x + ∆x)² + (x + ∆x) – (x² + x)
= x² + 2x ∆x + (∆x)² + x + ∆x – x² – x
= 2x ∆x + (∆x)² + ∆x
= 2(10) (0.1)+ (0.1)² +(0.1)
= 2 + 0.01 + 0.1
= 2.11.

Question 10.
Verify Rolle’s theorem for the function y = f(x) = x² – 1 on [-1, 1].
Solution:
Given y = f(x) = x² – 1
since f is a second degree polynomial
∴ f is continuous on [-1, 1] and f is derivable on (-1, 1)
Also f (-1) = (-1 )² – 1 = 1 – 1 = 0
f(1) = 1² – 1 = 1 – 1 = 0
∴ f(-1) = f(1)
∴ f statistics all the conditions of Rolle’s theorem.
∴ There exists c ∈ (-1, 1) such that f'(c) = 0.
f(x) = x² + x
⇒ f'(x) = 2x + 1
⇒ f'(x) = 2x + 1
⇒ f'(c) = 2c + 1
⇒ 2c = – 1
⇒ c = \(\frac{-1}{2}\) ∈ (-1, 1)
Hence Roll’s theorem is verified.

Section – B (5 × 4 = 20 Marks)

II. Short Answer Type Questions.

1. Attempt any five questions.
2. Each question carries four marks.

Question 11.
A(5, 3) and B(3, -2) are two fixed points. Find the equation of the locus of a P, so that the area of triangle PAB is 9.
Solution:
Given A = (5, 3)
B = (3, – 2)
Let P(x₁, y₁) be any point on the locus.
Given geometric condition is area of ∆PAB is 9 units,

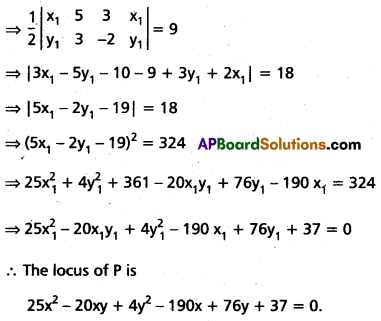
Question 12.
When the axes are rotated through an angle \(\frac{\pi}{4}\), find the transformed equation of 3x² + 10xy + 3y² = 9.
Solution:
Given equation is 3x² + 10xy + 3y² – 9 = 0

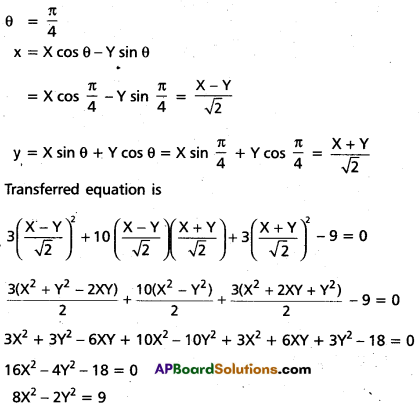
Question 13.
Transform the equation \(\frac{x}{a}+\frac{y}{b}\) = 1 into the normal form when a > 0 and b > 0. If the perpendicular distance of the straight line from the origin is p, deduce that \(\frac{1}{p^2}\) = \(\frac{1}{a^2}+\frac{1}{b^2}\).
Solution:
Given \(\frac{x}{a}+\frac{y}{b}\) = 1
⇒ \(\frac{b x+a y}{a b}\) = 1

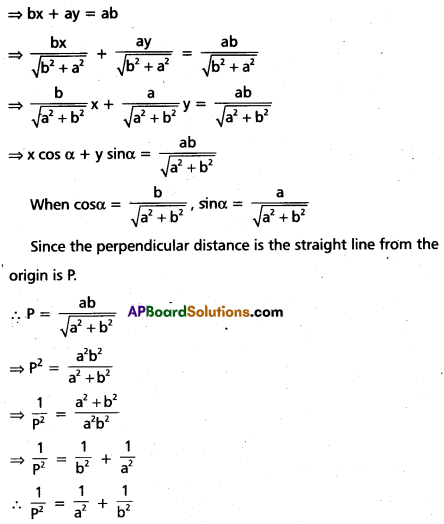
Question 14.
Check the continuity of the function f given by

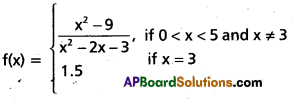
at the point 3.
Solution:
Given f(x) = \(\frac{x^2-9}{x^2-2 x-3}\), 0 < x < 5, x ≠ 3
= 1.5, x = 3

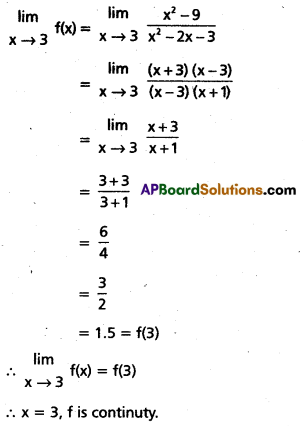
Question 15.
If y = tan^-1\(\left(\frac{2 x}{1-x^2}\right)\)(| x |< 1), then find \(\frac{d y}{d x}\).
Solution:
Given y = tan^-1 \(\left(\frac{2 x}{1-x^2}\right)\)(|x| < 1)
Put x = tan θ
⇒ θ = tan^-1x.
∴ y = tan^-1\(\left[\frac{2 \tan \theta}{1-\tan ^2 \theta}\right]\)
⇒ y = tan^-1 (tan 2θ)
⇒ y = 2θ
⇒ y = 2 tan^-1x.
Differentiating with respect to ‘x’ on both sides, we have
\(\frac{d y}{d x}\) = \(\text { 2. } \frac{1}{1+x^2}\)
\(\frac{d y}{d x}\) = \(\frac{2}{1+x^2}\)

Question 16.
Find the equations of tangent and normal to the curve x = cost, y = sint, at t = \(\frac{d y}{d x}\).
Solution:

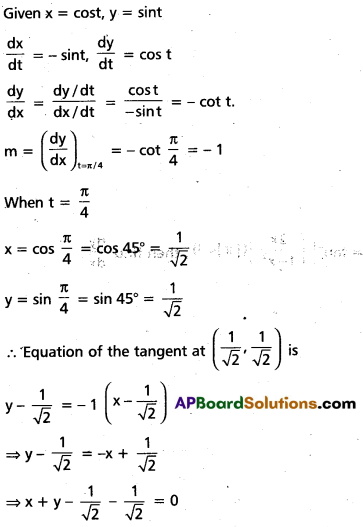
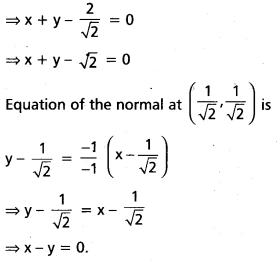
Question 17.
A stone is dropped into a quiet lake and ripples move in circles at the speed of 5cm/sec. At the instant when the radius of circular ripple is 8cm, how fast is the enclosed area increases ?
Solution:
Let r, A be the radius and area of circular ripple.
Given \(\frac{\mathrm{dr}}{\mathrm{dt}}\) = 5cm/sec and r = 8.
A = πr²
⇒ \(\frac{\mathrm{dA}}{\mathrm{dt}}\) = \(\pi .2 \mathrm{r} . \frac{\mathrm{dr}}{\mathrm{dt}}\)
when r = 8
\(\frac{\mathrm{dA}}{\mathrm{dt}}\) = π.2r.\(\frac{\mathrm{dr}}{\mathrm{dt}}\)
= 80 sq.cm/sec.

Section – C

III. Long Answer Type Questions.

1. Attempt any five questions.
2. Each question carries seven marks.

Question 18.
Find the orthocentre of the triangle whose vertices are (-2, -1) (6, -1) and (2, 5).
Solution:
Let A = (-2, -1); B = (6, -1); C = (2, 5)

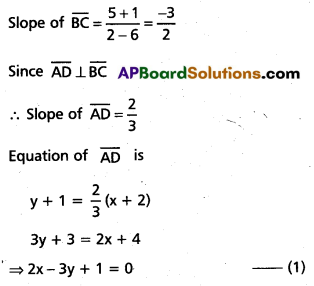
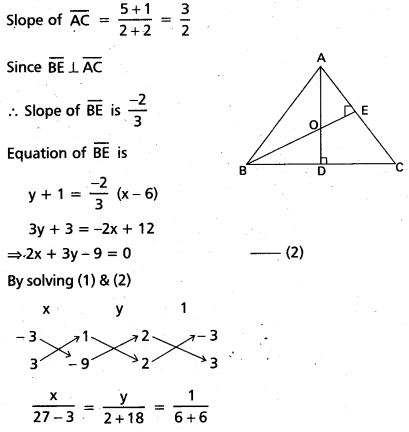
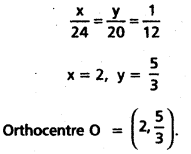
Question 19.
Show that the product of the perpendicular distances from the origin to the pair of straight lines represented by ax² + 2hxy + by² + 2gx + 2fy + c = 0 is \(\frac{|c|}{\sqrt{(a-b)^2+4 h^2}}\).
Solution:
Let S ≡ ax² + 2hxy + by² + 2gx + 2fy + c = 0
Let s = 0 represent pain of lines be
l₁x + m₁y + n₁ = 0 …… (1)
l₂x + m₂y + n₂ = 0 ……. (2)
∴ s ≡ (l₁x + n₁y + n₁) (l₂x + m₂y + n₂)
Comparing the co-efficients of like terms on both sides, we have
l₁l₂ = a; l₁m₁ = 2h
m₁m₂ = b; l₁n₂ + l₂n₁ = 2g
n₁n₂ = c; m₁n₂ + m₂n₁ = 2f
The perpendicular distance from origin to the line (1) = \(\frac{\left|\mathrm{n}_1\right|}{\sqrt{l_1^2+\mathrm{m}_1^2}}\)
The perpendicular distance from origin to the line (2) = \(\frac{\left|n_2\right|}{\sqrt{l_2^2+m_2^2}}\)
∴ The product of the perpendicular distances from origin to the pair of straigh lines represented by s = 0 is \(\frac{\left|n_1\right|}{\sqrt{l_1^2+m_1^2}} \cdot \frac{\left|n_2\right|}{\sqrt{l_2^2+m_2^2}}\)
= \(\frac{\left|n_1 n_2\right|}{\sqrt{\left(l_1^2+m_1^2\right)\left(l_2^2+m_2^2\right)}}\)

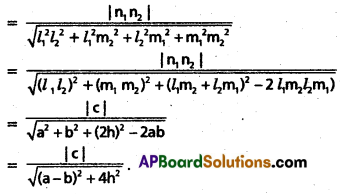
Question 20.
Find the condition for the fines joining the origin to the points of intersection of the circle x² + y² = a² and the line lx + my = 1 to coincide.
Solution:
Equation of the circle is x² + y² = a² ……… (1)
Equation of the line AB is lx + my = 1 ………. 2
Homogenising (1) with the help of (2)
∴ The combined equation of OA, OB is x² + y² = a² . 1²
= a² (lx + my)²
= a² (l²x² + 2lmxy + m²y²)
= a²l²x² + 2a²lmxy + a²m²y²
⇒ (a²l² – 1) x² + 2a² lmxy + (a²m² – 1) = 0
Since the lines OA, OB coincide.
∴ h² = ab
⇒ (a² lm)² = (a²l² – 1) (a²m² – 1)
⇒ a⁴l²m² = a⁴l²m² – a²l² – a²m² + 1
⇒ 0 = – a²l² – a²m² + 1
⇒ a²l² + a²m² = 1
⇒ a²(l² + m²) = 1.

Question 21.
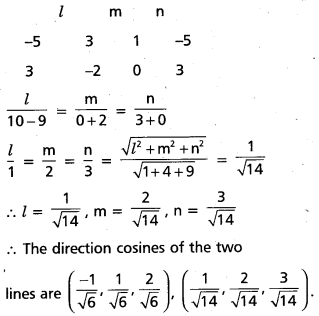
Find the direction cosines of two lines which are connected by the relations l – 5m + 3n = 0 and 7l² + 5m² – 3n² = 0.
Solution:
Given l – 5m + 3n = 0
⇒ l = 5m – 3n ……. (1)
7l² + 5m² – 3n² = 0
⇒ 7(5m – 3n)² + 5m² – 3n² = 0
⇒ 7(25m² – 30mn + 9n²) + 5m² – 3n² = 0
⇒ 175m² – 210mn + 63n² + 5m² – 3n² = 0
⇒ 180m² – 210mn + 60n² = 0
⇒ 6m² – 7mn + 2n² = 0
⇒ 6m² – 4mn – 3mn + 2n² = 0
⇒ 2m(3m – 2n) – n (3m – 2n) = 0
⇒ (2m – n) (3m – 2n) = 0
⇒ 2m – n = 0 ………. (2)
3m – 2n = 0 ….. (3)
Solving (1) and (2)

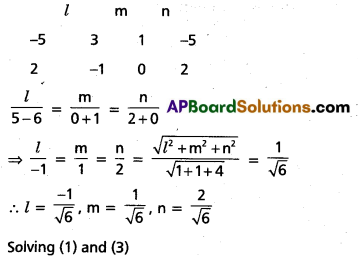
Question 22.
Find the derivative of the function (sinx)^logx + x^sinx.
Solution:
Given y = (sin x)^{log x} + x^{sin x}.
Let u = (sinx)logx
Taking logarithms on bothsides, we have
log u = log x. log (sin x)
Differentiating w.r.to ‘x’ on bothsides, we have

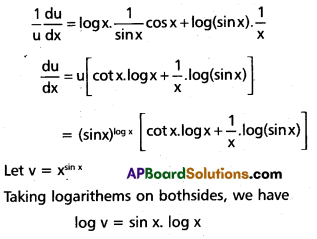
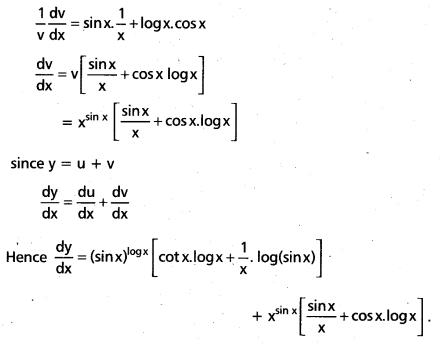
Differentiating w.r.to x on bothsides, we have

Question 23.
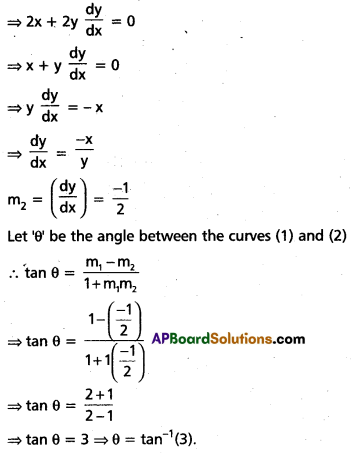
Find the angle between the curves, y² = 4x; x² + y² = 5.
Solution:
Given curve equations are
y² = 4x ……… (1)
x² + y² = 5 ……… (2)
From (1) and (2)
x² + 4x = 5 ⇒ x² + 4x – 5 = 0.
⇒ x² + 5x – x – 5 = 0
⇒ x (x + 5) – 1 (x + 5) = 0
⇒ (x – 1) (x + 5) = 0
⇒ x = 1, x = – 5
If x = 1 then y² = 4
⇒ y = ± 2.
∴ The points of intersection of the curves (1) and (2) are (1, 2) and (1, -2).
y² = 4x
Differentiating w.r.to ‘x’ on bothsides, we have

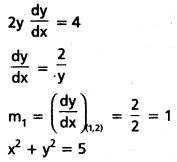
Differentiating w.r.to. ‘x’ on both sides, we have

Question 24.
A window is in the shape of a rectangle surrounded by a semicircle. If the perimeter of the window is 20 ft., find the maximum area.
Solution:
Let length of the rectangle be 2x and breadth by y and radius of the semi-circle in x.
∴ Perimeter = 20
⇒ y + 2x + y + πx = 20
⇒ 2x + 2y + πx = 20
⇒ 2y = 20 – 2x – πx
⇒ y = 10 – x – \(\frac{\pi}{2}\)x.

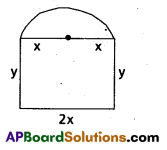
Window area = Area of the rectangle + Area of semi-circle