TS Inter 2nd Year Maths 2B Question Paper May 2017

Access to a variety of TS Inter 2nd Year Maths 2B Model Papers and TS Inter 2nd Year Maths 2B Question Paper May 2017 allows students to familiarize themselves with different question patterns.

TS Inter 2nd Year Maths 2B Question Paper May 2017

Section – A
(10 × 2 = 20)

Time : 3 Hours
Max. Marks : 75

Note : This question paper consists of three Sections – A, B and C.

Section – A

I. Very Short Answer Type Questions.

1. Attempt all questions.
2. Each question carries two marks.

Question 1.
Find the equation of a circle which is concentric with x² + y² – 6x – 4y – 12 = 0 and passing through (-2, 14).
Solution:
Let s ≡ x² + y² – 6x – 4y – 12 = 0
The equation of a circle which is concentric with s = 0 is x² + y² – 6x – 4y + k = 0 …….. (1)
If (1) passes through (-2, 14) then
4 + 196 + 12 – 56 + k = 0
⇒ 156 + k = 0
⇒ k = -156
Hence required circle equation is x² + y² – 6x – 4y – 156 = 0

Question 2.
Find the value of ‘k’, if the points (1, 3) and (2, k) are conjugate with respect to the circle x² + y² = 35.
Solution:
Let s ≡ x² + y² – 35 = 0
Since the points (1, 3) and (2, k) are conjugate with respect to the circle s = 0
∴ S₁₂ = 0
⇒ x₁ x₂ + y₁ y₂ – 35 = 0
⇒ 1(2) + 3(k) – 35 = 0
⇒ 3k – 33 = 0
⇒ 3k = 33
⇒ k = 11

Question 3.
Find the equation of the radical axis of the circles x² + y² – 3x – 4y + 5 = 0 and 3(x² + y²) – 7x + 8y – 11 = 0.
Solution:
Let s ≡ x² + y² – 3x – 4y + 5 = 0
S¹ = x² + y² – \(\frac{7}{3}\)x + \(\frac{8}{3}\)y – \(\frac{11}{3}\) = 0
The radical axis of the circles s = 0, s¹ = 0 is s – s¹ = 0

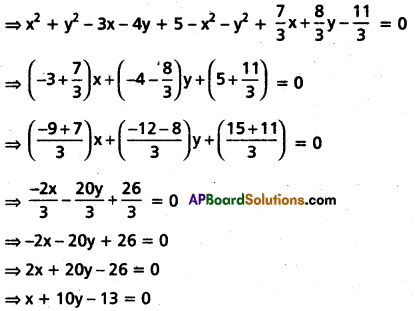
Question 4.
Find the co-ordinates of the points on the parabola y² = 8x whose focal distance is 10.
Solution:
Given parabola equation is y² = 8x
Hence 4a = 8 ⇒ a = 2
∴ Focus s = (2, 0)
Let p(x₁, y₁) be a point on the parabola
Given sp = 10

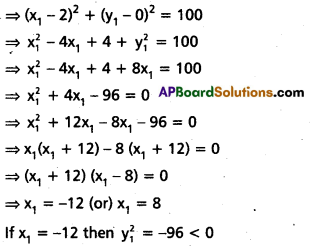
If x₁ = 8 then \(y_1^2\) = 64 ⇒ y₁ = ±8
∴ co-ordinates on the parabola are (8, 8), (8, -8)

Question 5.
If 3x – 4y + k = 0 is a tangent to the hyperbola x² – 4y² = 5, then find the value of ‘k’.
Solution:
Given hyperbola equation is x² – 4y² = 5
⇒ \(\frac{x^2}{5}\) – \(\frac{4 y^2}{5}\) = 1
⇒ \(\frac{x^2}{5}\) – \(\frac{y^2}{5 / 4}\) = 1 …… (1)
Here a² = 5, b² = \(\frac{5}{4}\)
Given line equation is 3x – 4y + k = 0
⇒ 4y = 3x + k
⇒ y = \(\frac{3}{4}\)k + \(\frac{k}{4}\) …….. (2)
Here m = \(\frac{3}{4}\), c = \(\frac{k}{4}\)
If (2) is a tangent to the hyperbola (1) then

Question 6.
Evaluate \(\int \sqrt{1-\sin 2 x} d x\) on I ⊂ \(\left[2 n \pi-\frac{3 \pi}{4}, 2 n \pi+\frac{\pi}{4}\right]\), n ∈ Z
Solution:

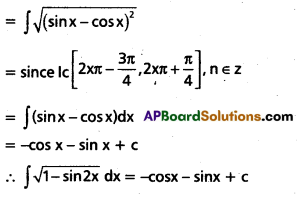
Question 7.
Evaluate \(\int \cos \sqrt{x}\) dx on R
Solution:
Let I = ∫cos\(\sqrt{x}\) dx
Let \(\sqrt{x}\) = t
Then \(\frac{1}{2 \sqrt{x}}\)dx = dt
⇒ dx = 2\(\sqrt{x}\) dt
⇒ dx = 2t dt

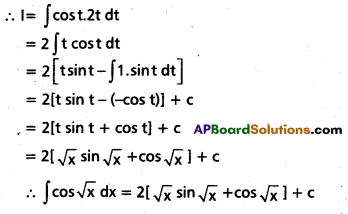
Question 8.
Evaluate \(\int_{-\pi / 2}^{\pi / 2} \frac{\cos \mathrm{x}}{1+\mathrm{e}^{\mathrm{x}}}\)dx
Solution:
Let I = \(\int_{-\pi / 2}^{\pi / 2} \frac{\cos x}{1+e^x} d x\) ………. (1)

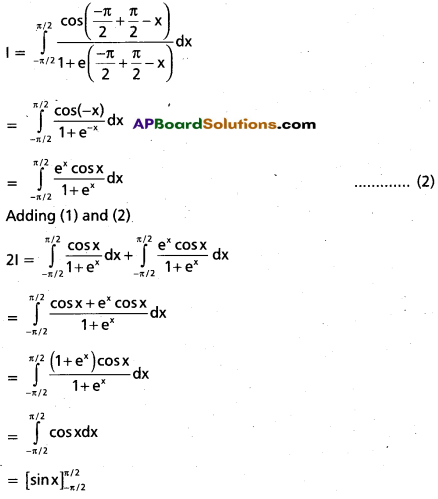
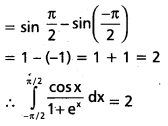
Question 9.
Find the area of the region enclosed by y = x³ + 3, y = 0, x = -1, x = 2.
Solution:
Area = \(\int_{-1}^2 y d x\)

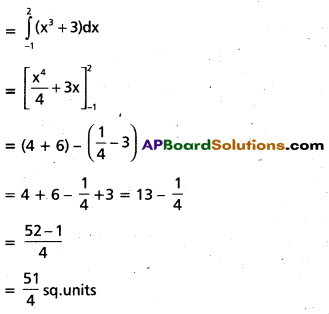
Question 10.
Find the order and degree of the differential equation
\(\left[\frac{d^2 y}{d x^2}+\left(\frac{d y}{d x}\right)^3\right]^{6 / 5}\) = 6y
Solution:
Order = 2
Degree = 1

Section – B

II. Short Answer Type Questions.

1. Attempt any five questions.
2. Each question carries four marks.

Question 11.
Find the equation of the tangent to x² + y² – 2x + 4y = 0 at (3, -1). Also find the equation of tangent parallel to it.
Solution:
Let s = x² + y² – 2x + 4y = 0
Center = (1, -2) = (-g, -f)
Radius r = \(\sqrt{1+4}\) = \(\sqrt{5}\)
The equation of the tangent to the circle s = 0 at (3, -1) is s₁ = 0
⇒ xx₁ + yy₁ – (x + x₁) + 2(y + y₁) = 0
⇒ x(3) + y(-1) – (x + 3) + 2(y – 1) = 0
⇒ 3x – y – x – 3 + 2y – 2 = 0
⇒ 2x + y – 5 = 0 ………. (1)
∴ Slope of the tangent = \(\frac{-2}{+1}\) = -2 = m
The equation on the tangent parallel to (1) is (y – f) = m(x – g) ±
r\(\sqrt{1+\mathrm{m}^2}\)
⇒ y+ 2 = -2(x – 1) ± \(\sqrt{5} \sqrt{1+4}\)
⇒ y + 2 = -2x + 2 ± 5
⇒ 2x + y = ± 5 ⇒ 2x + y – 5 = 0 and 2x + y + 5 = 0

Question 12.
Find the equation of the circle passing through the points of intersection of the circles x² + y² – 8x – 6y + 21 = 0, x² + y² – 2x – 15 = 0 and (1, 2).
Solution:
Let S ≡ x² + y² – 8y – 6y + 21 = 0
S¹ ≡ x² + y² – 2x – 15 = 0
S – S¹ = x² + y² – 8x – 6y + 21 – x² – y² + 2x + 15
= -6x – 6y + 36
The equation of the circle passing through the points of intersection of the circles s = 0, s¹ = 0 is
s + λ(s – s¹) = 0
⇒ (x² + y² – 8x – 6y + 21) + λ(-6x – 6y + 36) = 0
If this circle passing (1, 2) then
(1 + 4 – 8 – 12 + 21) + λ(-6 – 12 + 36) = 0
⇒ 6 + 18λ = 0
⇒ 18λ = -6
⇒ λ = \(\frac{-1}{3}\)
Hence required circle equation is
(x² + y² – 8x – 6y + 21) – \(\frac{1}{3}\)(-6x – 6y + 36) = 0
⇒ 3x² + 3y² – 24x – 18y + 63 + 6x + 6y – 36 = 0
⇒ 3x² + 3y² – 18x – 12y + 27 = 0
⇒ x² + y² – 6x – 4y + 9 = 0

Question 13.
If the normal at one end of a latus rectum of the ellipse \(\frac{x^2}{a^2}+\frac{y^2}{b^2}\) = 1 passes through one end of the minor axis, then show that e⁴ + e² = 1 (e = eccentricity of the ellipse).
Solution:
Given ellipse equation is \(\frac{x^2}{a^2}\) + \(\frac{y^2}{b^2}\) = 1
Let be the one end of the latus rectum.
∴ The equation of the normal at L is
\(\frac{a^2 x}{a e}\) – \(\frac{b^2 y}{b^2 / a}\) = a² – y²
⇒ \(\frac{a x}{e}\) – ay = a² – b² is a line
Passes through the one end B’ = (0, -b)
⇒ \(\frac{a(0)}{e}\)a(-b) = a² – b²
⇒ ab = a² – b²
⇒ ab = a² – a²(1 – e²)
⇒ ab = a²e²
⇒ e² = \(\frac{b}{a}\)
⇒ e⁴ = \(\frac{b^2}{a^2}\)
⇒ e⁴ = \(\frac{a^2\left(1-e^2\right)}{a^2}\)
⇒ e⁴ = 1 – e²
⇒ e⁴ + e² = 1

Question 14.
Find the eccentricity, length of latus rectum, foci and the equations of directrices of the ellipse 9x² + 16y² = 144.
Solution:
Given ellipse equation is 9x² + 16y² = 144

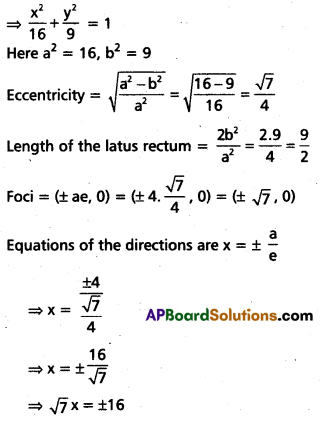
Question 15.
Prove that the point of intersection of two perpendicular tangents to the hyperbola \(\frac{x^2}{a^2}+\frac{y^2}{b^2}\) = 1 lies on the circle x² + y² = a² – b².
Solution:
Let p(x₁, y₁) be the point or intersection of two perpendicular tangents to the hyperbola \(\frac{x^2}{a^2}-\frac{y^2}{b^2}\) = 1
Equation or the tangent can be taken as y = mx ± \(\sqrt{a^2 m^2-b^2}\)
This tangent passes through (x₁, y₁)

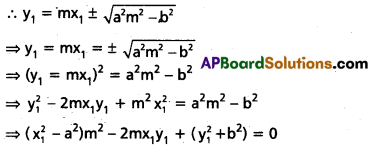
This is a quadratic equation in m giving the values set m₁, m₂ which are the slopes of the tangents passing through p.
Since tangents an perpendicular
∴ m₁m₂ = -1
⇒ \(\frac{y_1^2+b^2}{x_1^2-a^2}\) = -1
⇒ \(y_1^2\) + b² – \(\mathrm{x}_1^2\) + a²
⇒ \(\mathrm{x}_1^2\) + \(y_1^2\) = a² – b²
∴ The locus of P is x² + y² = a² – b².

Question 16.
Evaluate \(\int_{-a}^a x^2\left(a^2-x^2\right)^{3 / 2}\) dx.
Solution:
Let I = \(\int_{-a}^a x^2\left(a^2-x^2\right)^{3 / 2}\) dx
Since x²(a² – x²)^3/2 is an even function
\(2 \int_0^a x^2\left(a^2-x^2\right)^{3 / 2}\) dx
Put x = a sin θ
⇒ dx = a cosθ dθ
Equation or the tangent can be taken as
y = mx ± \(\sqrt{a^2 m^2-b^2}\)
This tangent passes through (x₁, y₁)

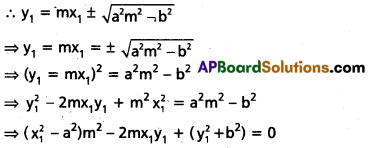
This is a quadratic equation in m giving the values set m₁, m₂ which are the slopes of the tangents passing through p.
Since tangents an perpendicular = -1
m₁m₂ = -1
⇒ \(\frac{y_1^2+b^2}{x_1^2-a^2}\) = 1
⇒ \(\mathrm{y}_1^2\) + b² – \(x_1^2\) + a²
⇒ \(\mathrm{x}_1^2\) + \(\mathrm{y}_1^2\) = a² – b²
∴ The locus of P is x² + y² = a² – b²

Or

Let I = \(\int_{-a}^a x^2\left(a^2-x^2\right)^{3 / 2} d x\)
Since x²(a² – x²)^3/2 is an even function
\(2 \int_0^a x^2\left(a^2-x^2\right)^{3 / 2}\) dx
Put x = a sin θ
⇒ dx = a cosθ dθ

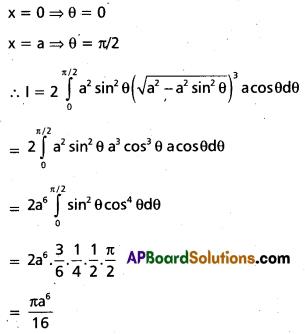
Question 17.
Solve the differential equation \(\frac{d y}{d x}\) = e^x-y + x²e^-y.
Solution:
Given differential equation is
\(\frac{d y}{d x}\) = e^x-y + x²e^-y
= e^x.e^-y + x²e^-y
⇒ \(\frac{d y}{e^{-y}}\) = (e^x + x²)dx
⇒ e^y dy = (e^x + x²)dx
integrating
∫e^ydy = ∫(e^x + x²)dx + c
⇒ e^y = e^x + \(\frac{x^3}{3}\) + c
Hence the general solution of (1) is
⇒ e^y = e^x + \(\frac{x^3}{3}\) + c

Section – C
(5 × 7 = 35)

III. Long Answer Type Questions.

1. Attempt any five questions.
2. Each question carries seven marks.

Question 18.
Find the equation of a circle passing through (2, -3) and (-4, 5) and having a centre on 4x + 3y + 1 =0.
Solution:
Let x² + y² + 2gx + 2fy + c = 0 …… (1)
be the required circle.
If (1) passes through (2, -3) then
4 + 9 + 4g – 6f + c = 0
4g – 6f + c = -13 …….. (2)
If (1) passes through (-4, 5) then
16 + 25 – 8g + 10f + c = 0
⇒ 8g – 10f – c = 41 …….. (3)
center (-g, -f) lies on 4x + 3y + 1 = 0
⇒ 4(-g) + 3(-f) + 1 = 0
⇒ -4y – 3f + 1 =0
⇒ 4g + 3f – 1 = 0 ……….. (4)
(2) + (3) ⇒ 12g – 16f = 28
⇒ 3g – 4f = 7
⇒ 3g – 4f – 7 = 0 …….. (5)
Solving (4) & (5)

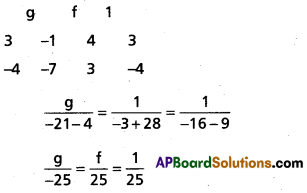
g = -1, f = 1
From (2) + 4 + 6 + c = – 13
⇒ c = -23
∴ g = -1, f = 1, c = -23
Hence required circle equation is
x² + y² – 2x + 2y – 23 = 0

Question 19.
Show that the circles x² + y² – 4x – 6y – 12 = 0 and 5(x² + y²) – 8x – 14y – 32 = 0 touch each other. Also find the point of contact and common tangent at this point of contact.
Solution:

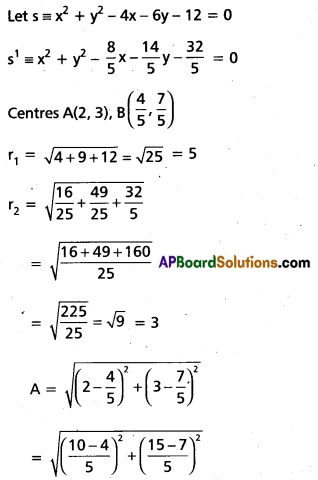
= \(\sqrt{\frac{36}{25}+\frac{64}{25}}\) = \(\sqrt{\frac{100}{25}}\) = \(\sqrt{4}\) = 2
∴ AB = r₁ – r₂
∴ The circles touch internally.
p divides AB externally in the ratio 5 : 3
p = \(\left(\frac{5 . \frac{4}{5}-3.2}{5-3}, \frac{5 . \frac{7}{5}-3.3}{5-3}\right)\)
= (-1, -1)
Equation of the tangent to the circle s = 0 at p is s₁ = 0.
⇒ x(x₁) + y(y₁) – 2(x + x₁) – 3(y + y₁) – 12 = 0
⇒ x(-1) + y(-1) – 2(x – 1) -3 (y – 1) – 12 = 0
⇒ -x – y – 2x + 2 – 3y + 3 – 12 = 0
⇒ -3x – 4y – 7 = 0
⇒ 3x + 4y + 7 = 0

Question 20.
Find the equation of the parabola whose axis is parallel to x – axis and which passes through the points (-2, 1), (1, 2) and (-1, 3).
Solution:
Since axis is parallel to x-axis
∴ Equation of the parabola is
x = ay² + by + c ………. (1)
since (1) passes through the points (-2, 1), (1,2), (-1,3)
-2 = a + b + c ……… (2)
1 = 4a + 2b + c ……… (3)
-1 = 9a + 3b + c …….. (4)

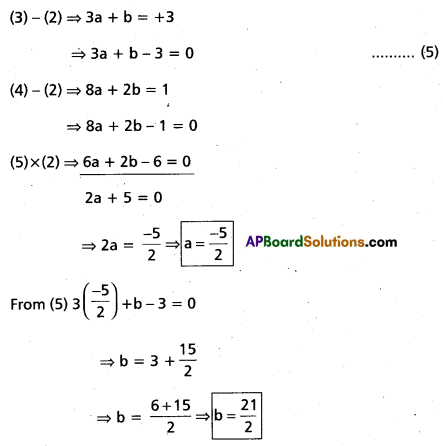
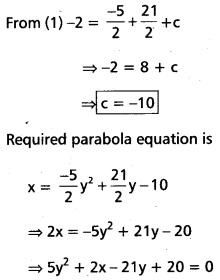
Question 21.
Evaluate \(\int \sqrt{\frac{5-x}{x-2}}\) dx on (2, 5).
Solution:

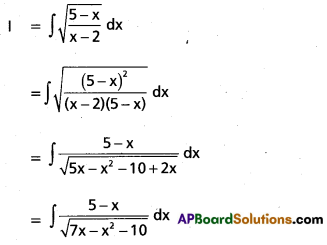
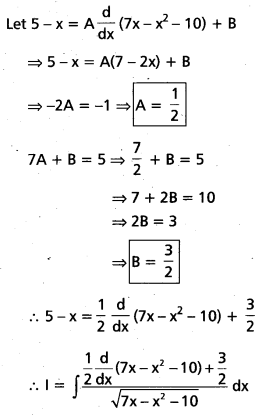
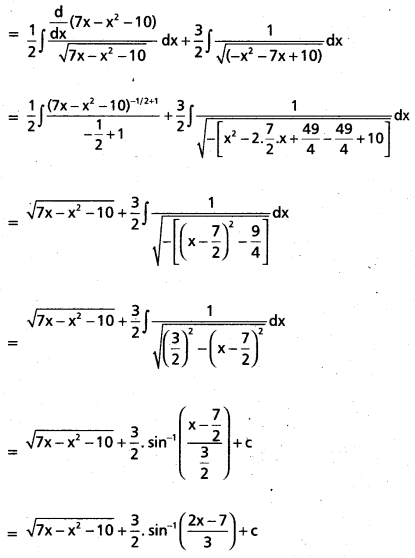
Question 22.
Obtain the reduction formula for ln = ∫Tanⁿx dx, n being a positive integer n ≥ 2 and deduce the value of ∫Tan⁶xdx.
Solution:

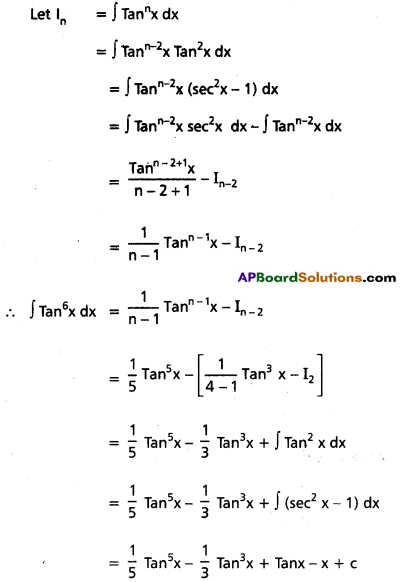
Question 23.
Evaluate \(\int_0^1 \frac{\log (1+x)}{1+x^2}\)dx.
Solution:
Put x = tan θ ⇒ dx = sec²θdθ
x = 0 ⇒ θ = 0
x = 1 ⇒ θ = \(\frac{\pi}{4}\)

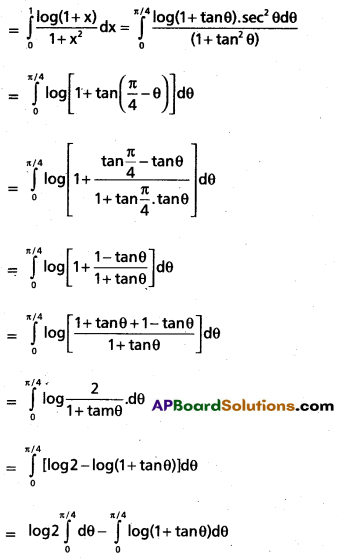
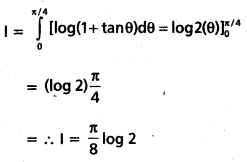
Question 24.
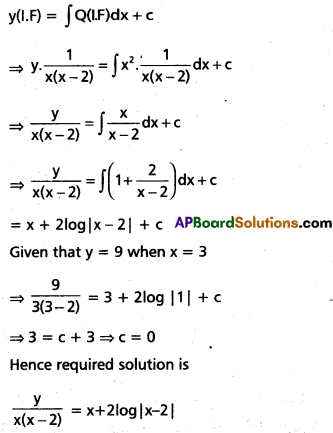
Find the solution of the equation
x(x – 2)\(\frac{\mathrm{dy}}{\mathrm{dx}}\) – 2(x – 1 )y = x³ (x – 2), which satisfies the condition that y = 9 when x = 3.
Solution:
Given differential equation is
\(\frac{\mathrm{dy}}{\mathrm{dx}}\) – \(\frac{2(x-1)}{x(x-2)}\)y = x²
This is a linear differential equation of first order in y.
Here p = \(\frac{-2(x-1)}{x(x-2)}\) and Q = x²

The general solution of (1) is