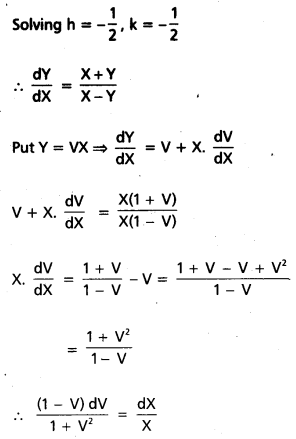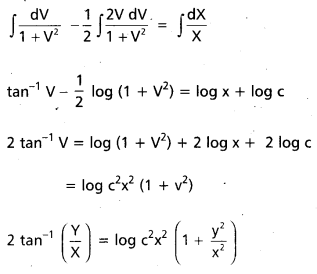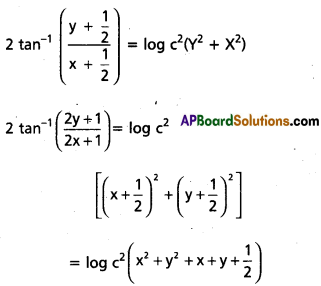TS Inter 2nd Year Maths 2B Question Paper March 2017

Access to a variety of TS Inter 2nd Year Maths 2B Model Papers and TS Inter 2nd Year Maths 2B Question Paper March 2017 allows students to familiarize themselves with different question patterns.

TS Inter 2nd Year Maths 2B Question Paper March 2017

Section – A
(10 × 2 = 20)

I. Very Short Answer Type questions.

1. Attempt all questions.
2. Each question carries two marks.

Question 1.
Obtain the parametric equation of the circle 4(x² + y²) = 9
Solution:
Equation of the circle is 4(x² + y²) = 9
x² + y² = 4
C (0, 0), r = \(\frac{3}{2}\)
Parametric equations are
x = \(\frac{3}{2}\) cos θ, y = \(\frac{3}{2}\) sin θ, 0 < θ < 2π

Question 2.
Find the value of k, if the points (4, 2) and (k, -3) are conjugate points with respect to the circle x² + y² – 5x + 8y + 6 = 0.
Solution:
Equation of the circle is x² + y² – 5x + 8y + 6 = 0
Polar of P(4, 2) is
x.4 + y.2 – \(\frac{5}{2}\) (x + 4) + 4 (y + 2) + 6 = 0
8x + 4y – 5x – 20 + 8y + 16 + 12 = 0
3x + 12y + 8 = 0
P(4, 2), Q(k, -3) are conjugate points
Polar of P passes through Q
∴ 3k – 36 + 8 = 0
3k = 28 ⇒ k = \(\frac{28}{3}\).

Question 3.
Find the angle between the circles given by the equations x² + y² – 12x – 6y + 41 = 0, x² + y² + 4x + 6y – 59 = 0.
Solution:
C₁ = (6, 3)
r₁ = (36 + 9 – 41)^1/2
r₁ = 2

C₂ = (6, 3)
r₂ = (4 + 9 + 59)^1/2
r₂ = (72)^1/2 = 6\(\sqrt{2}\)

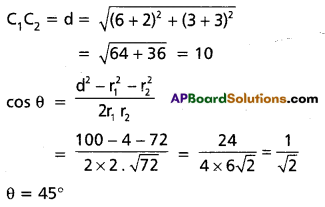
Question 4.
Find the coordinates of the points on the parabola y² = 8x whose focal distance is 10.
Solution:
Equation of the parabola is y² = 8x
4a = 8 ⇒ a = 2

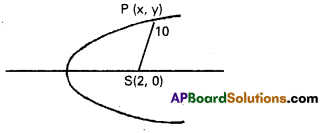
Co-ordinates of the focus S are (2, 0)
Suppose P(x, y) is the point on the parabola.
Given SP = 10 ⇒ SP² = 100
(x – 2)² + y² = 100
⇒ But y² = 8x
⇒ (x – 2)² + 8x = 100
⇒ x² – 4x + 4 + 8x – 100 = 0
⇒ x² + 4x – 96 = 0
⇒ (x+ 12) (x – 8) = 0
x + 12 = 0 or x – 8 = 0
x = -12, or 8

Case (i) x = 8
y² = 8.x = 8.8 = 64
y = ±8
Co-ordinates of the required points are (8, 8) and (8, -8)

Case (ii) x = -12
y² = 8(-12) = -96 < 0
y is not real.

Question 5.
If 3x – 4y + k = 0 is a tangent to the hyperbola x² – 4y² = 5 find the value of k.
Solution:
Equation of hyperbola x² – 4y² = 5
\(\frac{x^2}{5}\) + \(\frac{y^2}{\left(\frac{5}{4}\right)}\) = 1
a² = 5, b² = \(\frac{5}{4}\)
Equation of the given line is 3x – 4y + k = 0
4y = 3x + k
y = \(\frac{3}{4}\)x + \(\frac{k}{4}\)
m = \(\frac{3}{4}\) c = \(\frac{k}{4}\),
Condition for tangency is c² = a²m² – b²
\(\frac{k^2}{16}\) = 5.\(\frac{9}{16}\) – \(\frac{5}{4}\)
k² = 45 – 20 = 25
k = ±5.

Question 6.
Evaluate \(\frac{1}{\cosh x+\sinh x}\)dx on R.
Solution:
∫\(\frac{1}{\cosh x+\sinh x}\)dx
= ∫\(\frac{\cosh x-\sinh x}{\cosh ^2 x-\sinh ^2 x}\)dx
= ∫(coshx – sin hx)dx
= sinh x – coshx + C

Question 7.
Evaluate \(\frac{e^x(1+x)}{\cos ^2\left(x e^x\right)}\) dx on I ⊂ R \: {x ∈ R : cos (xe^x) = 0}
Solution:
t = x. e^x
dt = (x.e^x + e^x) dx = e^x(1 + x)dx
∫\(\frac{e^x(1+x)}{\cos ^2\left(x \cdot e^x\right)}\) = ∫\(\frac{d t}{\cos ^2 t}\) = ∫sec²t dt
= tan t + C
= tan(x.e^x) + C

Question 8.
Evaluate \(\int_{\frac{-\pi}{2}}^{\frac{\pi}{2}}\)sin|x|dx.
Solution:

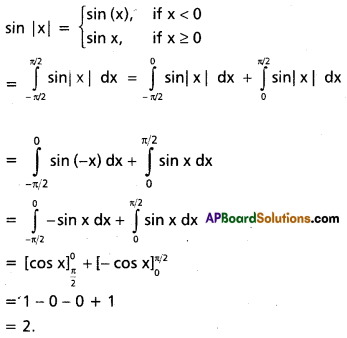
Question 9.
Evaluate \(\int_0^3 \frac{x}{\sqrt{x^2+16}}\) dx.
Solution:
x² + 16 = t²
2x dx = 2t dt
x dx = t dt

9 + 16 = t² ⇒ t = 5
0 + 16 = t² ⇒ t = 4
I = \(\int_4^5 \frac{\mathrm{tdt}}{\mathrm{t}}\)
= \(\int_4^5 \mathrm{dt}\)
= \([t]_4^5\)
= 5 – 4 = 1

Question 10.
Find the order of the differential equation of the family of all the circles with their centres at the origin.
Solution:
The family of all circles with their centres at the origin is x² + y² = r²
Here No. of arbitrary constants is one.
∴ order = 1.

Section – B
(5 × 4 = 20)

II. Short Answer Type questions.

1. Attempt any five questions.
2. Each question carries four marks.

Question 11.
If a point P is moving such that the lengths of tangents drawn from P to the circles x² + y² – 4x – 6y – 12 = 0 and x² + y² + 6x + 18y + 26 = 0 are in the ratio 2 : 3 then find the equation of the locus of P.
Solution:
P(x, y) is any point on the locus
S ≡ x² + y² – 4x – 6y – 12
PT₁ = \(²\)
S’ = x² + y² + 6x + 18y + 26
PT₂ = \(\sqrt{x^2+y^2+6 x+18 y+26}\)
Given \(\frac{\mathrm{PT}_1}{\mathrm{PT}_2}\) = \(\frac{2}{3}\)
⇒ \(\frac{\mathrm{PT}_1^2}{\mathrm{PT}_2^2}\) = \(\frac{4}{9}\)

9 P\(T_1^2\) = 4.P\(T_2^2\)
9(x² + y² – 4x – 6y – 12)
= 4(x² + y² + 6x + 18y + 26)
9x² + 9y² – 36x – 54y – 108
= 4x² + 4y² + 24x + 72y + 104
Locus of P is
5x² + 5y² – 60x – 126y – 212 = 0

Question 12.
Find the equation and the length of the common chord of the following circles:
x² + y² + 2x + 2y + 1 = 0; x² + y² + 4x + 3y + 2 = 0
Solution:
x² + y² + 2x + 2y + 1 = 0
x² + y² + 4x + 3y + 2 = 0
Equation of common chord is S – S’ = 0
(x² + y² + 2x + 2y + 1) – (x² + y² + 4x + 3y + 2) = 0
-2x – y – 1 = 0
2x + y + 1 = 0
Centre of circle is (-1, -1)
Radius = \(\sqrt{1+1-1}\) = 1
Length of ⊥ from centre (-1, -1) to the chord is

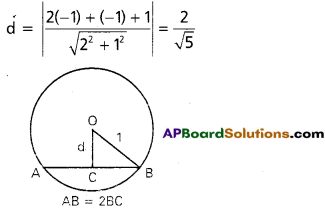
AB = 2\(\sqrt{r^2-d^2}\) = 2\(\sqrt{1-\frac{4}{5}}\)
AB = \(\frac{2}{\sqrt{5}}\)

Question 13.
Find the equation of ellipse in the standard form, if it passes through the points (-2, 2) and (3,-1).
Solution:
Equation of the ellipse in standard form is
\(\frac{x^2}{a^2}\) + \(\frac{y^2}{b^2}\) = 1
passes through (-2, 2); (3, -1)
\(\frac{4}{a^2}\) + \(\frac{4}{b^2}\) = 1 ………… (1)
\(\frac{9}{a^2}\) + \(\frac{1}{b^2}\) = 1 ………… (2)
Solving (i) and (ii) we get
\(\frac{1}{a^2}\) = \(\frac{3}{32}\) ; \(\frac{1}{b^2}\) = \(\frac{5}{32}\)
\(\frac{3 x^2}{32}\) + \(\frac{5 y^2}{32}\) = 1
3x² + 5y² = 32.

Question 14.
Find the equation of the tangents to the ellipse 2x² + y² = 8 which are
(i) parallel to x – 2y – 4 = 0,
(ii) perpendicular to x + y + 2 = 0
Solution:
i) Parallel to x – 2y – 4 = 0
Slope will be : \(\frac{1}{2}\)
Equation of tangent y = mx ± \(\sqrt{a^2 m^2+b^2}\)
y = \(\frac{1}{2}\)x ± \(\sqrt{a^2\left(\frac{1}{2}\right)^2+b^2}\)
\(\frac{x^2}{4}\) + \(\frac{y^2}{8}\) = 1
y = \(\frac{1}{2}\)x ± \(\sqrt{4 \times \frac{1}{4}+8}\)
y = \(\frac{1}{2}\)x ± 3
2y – x ± 6 = 0 required equation of tangents.
x – 2y ± 6 = 0.

ii) Perpendicular to x + y + 2 = 0
Slope of tangent be ‘1’ as it is perpendicular to above line
y = mx ± \(\sqrt{a^2 m^2+b^2}\)
y = x ± \(\sqrt{4+8}\)
y = x ± 2\(\sqrt{3}\)
⇒ x – y ± 2\(\sqrt{3}\) = 0.

Question 15.
If e, e₁ are the eccentricities of a hyperbola and its conjugate hyperbola prove that \(\frac{1}{e^2}\) + \(\frac{1}{\mathrm{e}_1^2}\) = 1.
Solution:

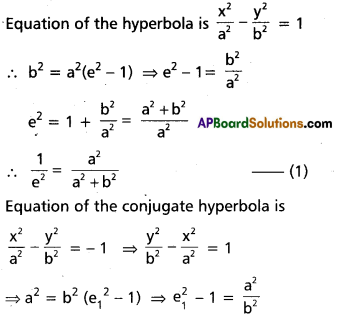
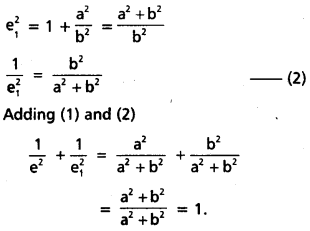
Question 16.
Find the area of the region bounded by the parabolas y² = 4x and x² = 4y.
Solution:

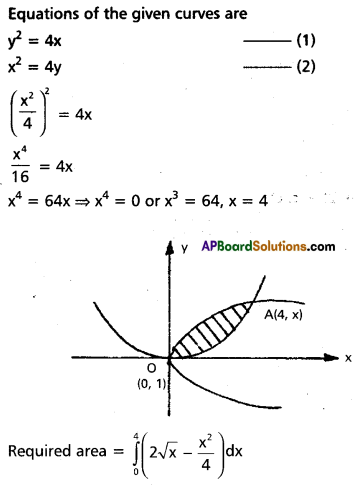
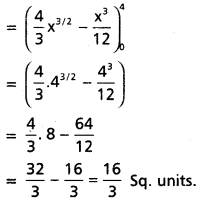
Question 17.
Solve the following differential equation (x + y + 1)\(\frac{d y}{d x}\) = 1
Solution:
\(\frac{d x}{d y}\) = x + y + 1
\(\frac{d x}{d y}\) = y + 1
I.F. = \(\mathrm{e}^{\int-d y}\) = e^-y
x.e^-y = ∫e^-y(y + 1)dy
= -(y + 1). e^-y + ∫e^-y. dy
= -(y + 1)e^-y – e^-y
= -(y + 2)e^-y + c
x = -(y + 2) + c.e^y

Section – C

III. Long Answer Type questions.

1. Attempt any five questions.
2. Each question carries seven marks.

Question 18.
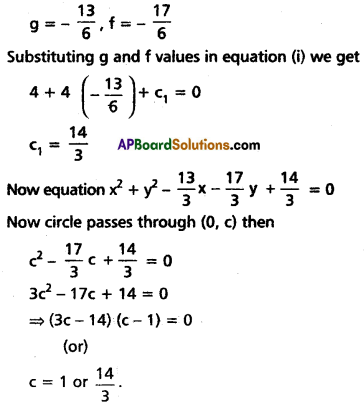
If (2, 0), (0, 1), (4, 5) and (0, c) are concyclic then find c.
Solution:
x² + y² + 2gx + 2fy + c₁ = 0
Satisfies (2, 0), (0, 1) (4, 5) we get
4 + 0 + 4g + c₁ = 0 ……. (1)
0 + 1 + 2g. 0 + 2f + c,sub>1 = 0 ……. (ii)
16 + 25 + 8g + 10f + c₁ = 0 …….. (iii)
(ii) – (i) we get
-3 – 4g + 2f = 0
4g – 2f = -3 …….. (iv)
(ii) – (iii) we get
-40 – 8g – 8f = 0 (or)
g + f = -5 ……. (v)
Solving (iv) and (v) we get

Question 19.
Find the transverse common tangents of the circles x² + y² – 4x – 10y + 28 = 0 and x² + y² + 4x – 6y + 4 = 0.
Solution:

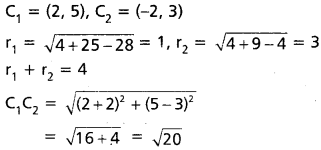
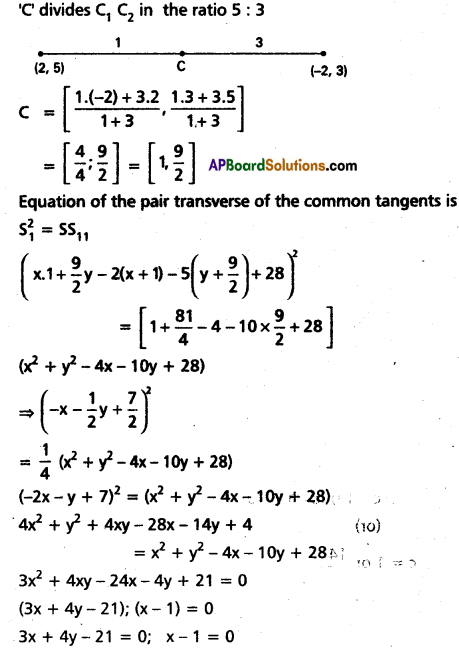
Question 20.
Derive the equation of a parabola in the standard form y² = 4ax with diagram.
Solution:
Equation of a parabola in standard form :
To study the nature of the curve, we prefer its equation in the simplest possible form. We proceed as follows to derive such an equation.

Let S be the focus, l be the directrix as shown in fig. Let z be the projection of ‘S’ on l and ‘A’ be the midpoint of \(\overline{S Z}\). A lies on the parabola because SA = AZ. A is called the vertex of the parabola. Let \(\overline{\mathrm{YAY}}\)‘ be the straight line through A and parallel to the directrix. Now take ZX as the X-axis and YY’ as the Y- axis.
Then A is the origin (0, 0). Let S = (a, 0), (a > 0). Then z = (-a, 0) and the equation of the directrix is x + a = 0.

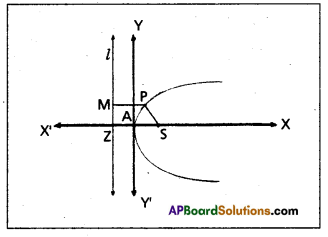
If P(x, y) is a point on the perpendicular and PM is the perpendicular distance from P to the directrix l, then \(\frac{S P}{S M}\) = e = 1
∴ (SP)² = (PM)²
(x – a)² + y² = (x + a)²
∴ y² = 4ax
Conversely if P(x, y) is any point such that y² = 4ax then
SP = \(\sqrt{(x-a)^2+y^2}\) = \(\sqrt{x^2+a^2-2 a x+4 a x}\) = \(\sqrt{(x+a)^2}\)
= |x + a| = PM
Hence P(x, y) is on the locus. In other words, a necessary and sufficient condition for the point P(x, y) to be on the parabola is that y² = 4ax.
Thus the equation of the Parabola is y² = 4ax.

Question 21.
Evaluate \(\int \frac{9 \cos x-\sin x}{4 \sin x+5 \cos x}\)dx.
Solution:

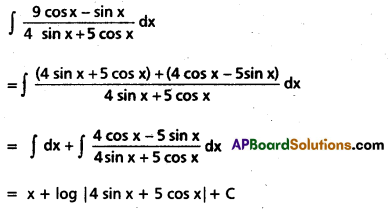
Question 22.
If I_n = ∫cosⁿ x dx, then show that I_n = \(\frac{1}{n}\)cos^n-1 x sin x + \(\frac{n-1}{n}\)I_n-2 and for n > 2 deduce the value of ∫cos⁴ x dx.
Solution:
I_n = ∫cosⁿx dx = ∫cos^n-1x. cos x dx
= cos^n-1x. sin x – ∫sin x. (n – 1) cos^n-2x (-sin x )dx
= cos^n-1x. sin x + (n – 1) + ∫cos^n-2x(1 – cos²x)dx
= cos^n-1x. sin x + (n – 1)I_n-2 – (n – 1) I_n
∴ I_n(1 + n – 1) = cos^n-1x. sinx + (n – 1)I_n-2
I_n = \(\frac{\cos ^{n-1} x \sin x}{n}\) + \(\frac{n-1}{n}\)I_n-2

Question 23.
Show that \(\int_0^{\frac{\pi}{2}} \frac{x}{\sin x+\cos x}\) dx = \(\frac{\pi}{2 \sqrt{2}}\) log (\(\sqrt{2}\) + 1).
Solution:

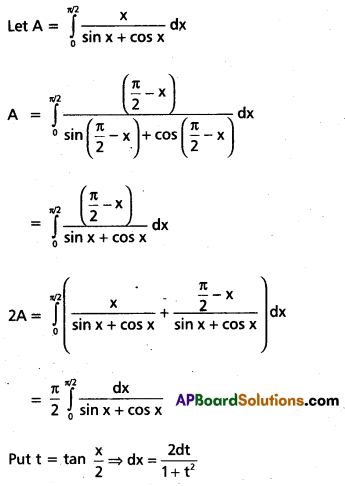
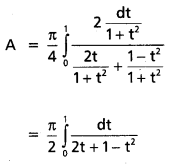
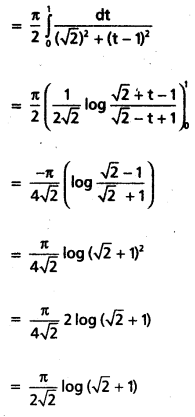
Question 24.
Solve the differential equation (x – y) dy = (x + y + 1)dx.
Solution: