TS Inter 1st Year Physics Question Paper March 2018

Thoroughly analyzing TS Inter 1st Year Physics Model Papers and TS Inter 1st Year Physics Question Paper March 2018 helps students identify their strengths and weaknesses.

TS Inter 1st Year Physics Question Paper March 2018

Time: 3 Hours
Maximum Marks: 60

Section – A (10 × 2 = 20)

Note :

• Answer all questions.
• Each question carries two marks.
• All are Very Short Answer Type Questions.

Question 1.
What is the Raman effect ?
Answer:
In elastic scattering of light by molecules. This is called Raman effect.

Question 2.
What are significant numbers ? Write the number of significant digits in the measurement of 0.002308.
Answer:
Significant numbers :

1. The digits of a number that are definitely known plus one more digit that is estimated are called significant numbers.
2. 0.002308 have 4 significant digits.

Question 3.
If |\(\vec{a}\) + \(\vec{b}\)| = |\(\vec{a}\) – \(\vec{b}\)|, what is the angle between \(\vec{a}\) and \(\vec{b}\) ?
Answer:
|\(\vec{a}\) + \(\vec{b}\)| = |\(\vec{a}\) – \(\vec{b}\)|
a² + b² + 2ab cos θ = a² + b² – 2ab cos θ
4ab cos θ = 0
cos θ = 0
θ = 90°

Question 4.
Can the coefficient of friction be greater than one ?
Answer:
Yes, coefficient of friction may be greater than one. In some particular cases it is possible.
They are :

1. Due to increase the inner molecular attractive forces between surfaces when the contact surfaces are highly polished.
2. When the contact surfaces of the bodies are inter locking the coefficient friction may be greater than one.

Question 5.
What is the principle behind the carburetor of an automobile ?
Answer:
The carburetor of automobile has a venturi channel (nozzle) through which air flows with a large speed. The pressure is then lowered at the narrow neck and the petrol is sucked up in the chamber to provide the correct mixture of air to fuel necessary for combustion.

Question 6.
If the diameter of a soap bubble is 10 mm and its surface tension is 0.04 N/m, find the excess pressure inside the bubble.
Answer:
D = 100 m
r = \(\frac{D}{2}\) = 5 mm = 5 × 10^-3 m
S = 0.04 N/m
P_i – P₀ = \(\frac{4 \times 0.04}{5 \times 10^{-3}}\) = 0.032 × 10³
P_i – P₀ = 32 N/m² (or) Pascal.

Question 7.
State Newton’s law of cooling.
Answer:
The rate of cooling of a body is directly proportional to the difference in the temperature of the body and its surroundings.
\(\frac{\mathrm{dQ}}{\mathrm{dt}}\) ∝ (T_B – T_S).

Question 8.
Why utensils are coated black ? Why the bottom of the utensils are made of copper ?
Answer:

1. Utensils are coated black, because it is a good absorber of heat.
2. Copper is a good conductor of heat. So, copper is used at the bottom of cooking utensils.

Question 9.
State Dalton’s law of partial pressures.
Answer:
The total pressure exerted by a mixture of non-reacting gases occupying a vessel is equal to the sum of the individual pres-sures which each gas would exert if it alone occupied the whole vessel, i.e., P = P₁ + P₂ + ……..

Question 10.
What is the law of equipartition of energy?
Answer:
Law of equipartition of energy : The total energy is equally distributed in all possible energy modes, with each mode having an average energy equal to \(\frac{1}{2}\)k_BT. This is known as the law of equipartition of energy.

Section – B (6 × 4 = 24)

Note :

• Answer any six of the following questions.
• Each question carries four marks.
• All are Short Answer Type Questions.

Question 11.
A man runs across the roof of a tall building and jumps horizontally on to the (lower) roof of an adjacent building. If his speed is 9 m/s and the horizontal distance between the building is 10 m and the height difference between the roofs is 9 m, will he be able to land on the next building ? (Take g = 10 m/s²)
Answer:

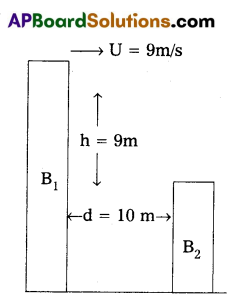
U = 9 m/s
h = 9 m
d = 10 m
g = 10 m/s²
Time of descent (t_d) = \(\sqrt{\frac{2 \mathrm{~h}}{\mathrm{~d}}}\)
Maximum horizontal distance
= U × \(\sqrt{\frac{2 \mathrm{~h}}{\mathrm{~g}}}\)
D = 9\(\sqrt{\frac{2 \times 9}{10}}\)
D = 12.07 m
But d = 10 m
∴ D > d
Person can safely land on next building.

Question 12.
Show that the trajectory of an object thrown at certain angle with the horizontal is a parabola.
Answer:

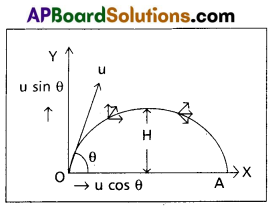
Consider a body is projected with an initial velocity (u) making an angle θ with the horizontal. The body does not experience acceleration in horizontal direction.

The velocity of the projectile can be resolved in to (i) u cos θ, horizontal component (ii) u sin θ, vertical component. The horizontal component of velocity remains constant through out the- motion. Only its veritcal component changes due to acceleration due to gravity (g).

The distance travelled along OX in time t is given by
x = u cos θ × t; t = \(\frac{\mathrm{x}}{\mathrm{u} \cos \theta}\) ________ (1)
The distance travelled along oy in time t is given by
y = (u cos θ) t \(\frac{1}{2}\) gt² ________ (2)
Substituting eq. (1) in eq. (2)
y = (u sin θ) \(\frac{\mathrm{x}}{\mathrm{u} \cos \theta}\) – \(\frac{1}{2}\) g (\(\frac{x^2}{u^2 \cos ^2 \theta}\)) [∵ u = u sin θ, a = -g, s = y]
y = x tan θ – (\(\frac{g}{2 u^2 \cos ^2 \theta}\)) x²
Let A = tan θ and B = \(\frac{g}{2 u^2 \cos ^2 \theta}\)
Y = Ax – Bx²
Where A and B are constants.
This is the equation of parabola.
∴ The trajectory of a projectile is parabola.

Question 13.
State Newton’s second law of motion. Hence derive the equation of motion F = ma from it.
Answer:
Newton’s second law of motion : “The rate of change of momentum of a body is directly proportional to the external force applied and takes place in the same direction”.

To Show F = ma : Let a body of mass ‘m’ moving with velocity ‘v’ under the action of an external force F in the direction of velocity.
Momentum ‘P’ of a body is the product of the mass and velocity v.
∴ P = mv ________ (1)
According to Newton’s Second Law of motion, we have dp
\(\frac{\mathrm{dp}}{\mathrm{dt}}\) ∝ F, F = External force
or F = k \(\frac{\mathrm{dp}}{\mathrm{dt}}\) ________ (2)
From equations (1) and (2) we have
F = K\(\frac{\mathrm{d}(\mathrm{mv})}{\mathrm{dt}}\) = K.m \(\frac{\mathrm{dv}}{\mathrm{dt}}\) = Kma ________ (3)
Since the rate of change of velocity \(\frac{\mathrm{dv}}{\mathrm{dt}}\) is the acceleration ‘a’ of the body.
In SI system the unit of force is Newton and is defined as that force which when action on a body of mass 1kg produces in it an acceleration of 1 ms^-2,
i.e., from equation (3),
If F = 1, m = 1 and a = 1, we get k = 1
Hence F = \(\frac{\mathrm{dp}}{\mathrm{dt}}\) = ma
∴ F = ma

Question 14.
Find the centre of mass of three particles, 100 gm, 150 gm and 200 gm placed at the vertices of an equilateral triangle of each side 0.5 m long. (Take 100 gm at origin and 150 gm along X-axis).
Answer:
With the x – and y – axis chosen as shown Figure, the coordinates of points O, A and B forming the wquilateral triangle are respectively (0, 0) (0.5, 0), (0.25, √3)- Let the masses 100g, 150g and 200g be located at O, A and B be respectively, then,

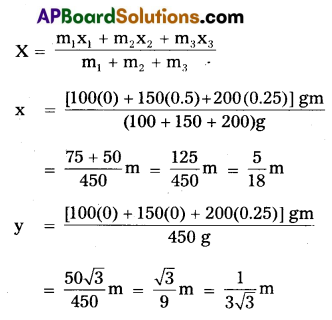
Question 15.
Define angular acceleration and torque. Obtain the relation between them.
Answer:
Angular acceleration (α) : The rate of change of angular velocity is called angular acceleration.
α = \(\frac{\omega}{t}\) (or) \(\frac{d \omega}{d t}\)

Torque (τ) : Torque is defined as the product of force and perpendicular distance of the line of action of the force from the point.
\(\vec{\tau}\) = \(\vec{r}\) × \(\vec{F}\) = rF sin θ

Relation between angular acceleration of torque :
Torque (τ) = Perpencicular distance × Force
\(\vec{\tau}\) = \(\vec{r}\) × \(\vec{F}\)
τ = r × ma (∵ F = ma)
τ = ma (rα) (∵ a = ra)
τ = mr² α
τ = Iα

Question 16.
What is a geostationary satellite ? State its uses.
Answer:
Geo-stationary satellite : If the period of revolution of an artificial satellite is equal to the period of rotation of earth, then such a satellite is called geo-stationary satellite. Time period of geo-stationary satellite is 24 hours.

Uses:

1. Study the upper layers of atmosphere.
2. Forecast the changes in atmosphere.
3. Know the shape and size of the earth.
4. Identify the minerals and natural resources present in¬side and on the surface of the earth.

Question 17.
Describe the behaviour of a wire under gradually increasing load.
Answer:
When the load is increased in steps, a graph is drawn between stress on y-axis and corresponding strain on x-axis.

1. Proportionality limit : In the linear position OA, stress is proportional to strain, i.e., Hookes law is obeyed by the wire upto point A. The graph is a straight line. When ever the stretching force at A is removed, the wire regains its original length.

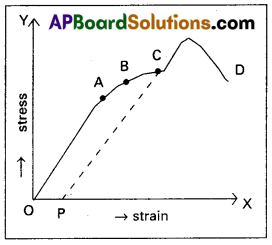
A is called proportionality limit.

2. Elastic limit: In the graph B is the elastic limit. Through the wire doesnot obey Hooke’s law at B. The wire regains its original length after removing the stretching force at B up to point B the wire is under elastic behaviour.

3. Permanent set (or) yield point : In the graph c is the yield point. If the stretching force at c is removed, the wire doesnot regain its original length and the length of the wire changes permanently. In this position the wire flows like a viscous liquid. After the point c, the wire is under plastic behaviour, c is called permanent set (or) yield point.

4. Breaking point : When the stress increased, the wire becomes thinner and thinner. When the stress increases to a certain limit the wire breaks. The stress at which the wire breaks is called breaking stress and the point D is called breaking point.

5. Elastic fatigue : The state of temporary loss of elastic nature of a body due to continuous strain is called elastic fatigue. When a body is subjected to continuous strain with in the elastic limit, it appears to have lost elastic property temporarily to some extent and becomes weak.

Question 18.
Explain conduction, convection and radiation with examples.
Answer:
The heat is transmitted in three types.
They are
1) Conduction
2) Convection
3) Radiation.

1) Conduction : The process of transmission of heat from one place to other without actual movement of the particles of the medium is called conduction.
Ex: When long iron rod is heated at one end, heat transmits to the other end.

2) Convection : The process of transmission of heat from one place to another by the actual movement of the particles is called convection.
Ex : If water in a beaker is heated, the particles of water at the bottom receive the heat first. These particles expand, become lighter and rise up. At the same time colder and denser particles reach the bottom. They get in their turn heated and move up. This process is known as convection.

3) Radiation: The process of transmission of heat from one place to another without any intervening medium is called radiation.
Ex : Earth receives heat radiations from the sun.

Section – C (2 × 8 = 16)

Note :

• Answer any two of the following questions.
• Each question carries eight marks.
• All are Long Answer Type Questions.

Question 19.
What are collisions ? Explain the types of collisions. Show that in the case of one dimensional elastic collision, the relative velocity of approach of two colliding bodies before collision is equal to the relative velocity of separation after collision. A body freely falling from a certain height ‘h’ after striking a smooth floor rebounds to a height h/2. What is coefficient of restitution between the floor and the body ?
Answer:
Collisions : A strong interactions between bodies that occurs for a very short interval during which redistribution of momenta occur ignoring the effect of other forces are called collisions.

Collisions are of two types :

1. Elastic collision : The collision in which both momentum and kinetic energy is constant is called elastic collision.
2. Inelastic collision : The collision in which momentum remains constant but not kinetic energy is called inelastic collision.

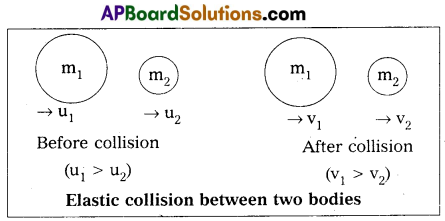
One dimensional elastic collision: Consider two spheres (bodies) which have smooth, non-rotating of masses m₁ and m₂ (m₁ > m₂) are moving along the straight line joining their centres of mass with initial velocities u₁ and u₂ (u₁ > u₂). They undergo head on collision and move along the same line after collision with final velocities v₁ and v₂. These two bodies exert forces on each other during collision.

Hence both momentum and kinetic energy are conserved. According to law of conservation of linear momentum.
m₁u₁ + m₂ u₂ = m₁ v₁ + m₂ v₂;
m₁ (u₁ – v₁) = m₂ (v₂ + u₂) ……. (1)
According to law of conservation of kinetic energy

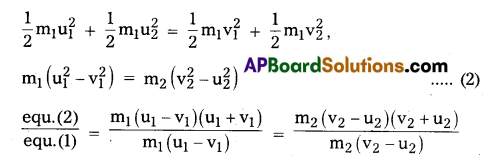
u₁ + v₁ = v₂ + u₂, u₁ – u₂ = v₂ = v₁ ……… (3)

From the above equation, in one dimensional elastic collision, the relative velocity of approach before collision is equal to the relative velocity of separation after collision.

From the above equation, in one dimensional elastic collision, the relative velocity of approach before collision is equal to the relative velocity of separation after collision.
From equation (3) u₁ – u₂ = v₂ – v₁, v₂ = u₁ – u₂ + v₁ ……….. (4)
Sub. equation (4) in equation (1) we get

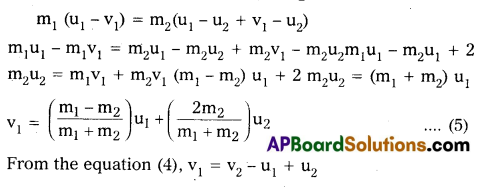
Sub. this value in equation (1) we get

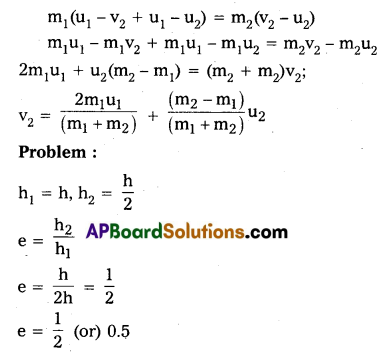
Question 20.
Show that the motion of a simple pendulum is simple harmonic and hence derive an equation for its time period. Calculate the change in the length of a simple pendulum of length 1 m, when its period of oscillation changes from 2 sec to 1.5 sec.
Answer:

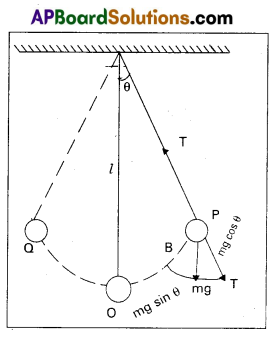
• Consider simple pendulum, a small bob of mass m tied to an inextensible mass less string of length L and other end of the string is fixed from a rigid support.
• Once the bob is slightly displaced and released, the begins to oscillate about mean position.
• Let θ be the angular displacement and T be the tension in the string.
• The forces acting on the bob are
  (a) tension T along the string
  (b) weight mg acts vertically downwards.
• The force mg can be resolved into two components
  1. mg cos θ along the PA and
  2. mg sin θ acts along PB.
• From figure at point P, T = mg cos θ ……….. (1)
• The force mg sin θ, w provide the restorin torque, which tends to bring the bob back to i mean position O.
• The restoring torque given by
  τ = Restoring force × ⊥^lar distance
  τ = – mg sin θ × L ………… (2)
  Here, negative sign shows that the torque acts to reduce θ.
  Then sin θ is replaced by θ i.e., sin θ ≈ θ; t = -mg Lθ …… (3)
  (∵ sin θ = θ – \(\frac{\theta^3}{3 !}\) + \(\frac{\theta^5}{5 !}\) …… )
• From equation (3), we note that τ ∝ θ and this τ will bring the bob back towards its equilibrium position.
  So, if the bob is left free, it will execute angular simple harmonic motion. Comparing equation (3), with the equation τ = -kθ, we have spring factor, k = mgl.
• Here inertia factor = Moment of inertia of the bob about the point of suspension = mL².
• In S.H.M, Time period (T) = 2π\(\sqrt{\frac{\text { Inertia factor }}{\text { Spring factor }}}\)
  T = 2π\(\sqrt{\frac{\mathrm{mL}^2}{\mathrm{mgL}}}\); T = 2π\(\sqrt{\frac{L}{g}}\)

Problem :
\(\frac{l_2}{l_1}\) = \(\frac{\mathrm{T}_2^2}{\mathrm{~T}_1}\)
l₂ = 1 × \(\frac{2.25}{4}\)
l₂ = 0.5625
Change in length = l₁ – l₂ = 1 – 0.5625
l₁ – l₂ = 0.4375 m

Question 21.
State second law of thermodynamics. How is heat engine different from a refrigerator ?
Answer:
1) Kelvin – Planck statement: “No process is possible whose sole result is the absorption of heat from a reservoir and the complete conversion of the heat into work”.
(or)
“It is impossible to derive a continuous supply of engines in cooling a body below the coldest of its surroundings”.

2) Clausius statement : “No process is possible whose sole result is the transfer of heat from a colder object to a hotter object”.
(or)
“Heat can not itself flow from cold body to hot body”. Heat engine: A device which converts heat energy into work is called heat engine.
A heat engine consists of the essential parts.

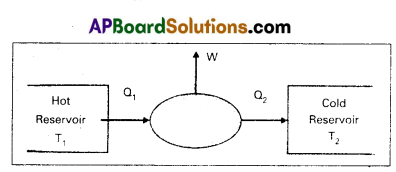
1. Source or hot reservoir : It is maintained at a very high temperature T₁ Heat is extracted from this body.
2. Working substance: In a steam engine working substance is steam. In diesel engine working substance is mixture of fuel vapour and air.
3. Sink or cold reservoir : It is maintained at a very low temperature T₂. It absorbs heat energy, rejected by working substance.

Work done : The difference of heat absorbed from source and heat rejected to sink is equal to work done by the engine. i.e., W = Q₁ – Q₂.
Efficiency : The ratio of workdone (W) by the engine to the amount of heat absorbed (Q₁) by the engine is called efficiency.
i.e., η = \(\frac{\mathrm{W}}{\mathrm{Q}_1}=\frac{\mathrm{Q}_1-\mathrm{Q}_2}{\mathrm{Q}_1}\) = 1 – \(\frac{\mathrm{Q}_2}{\mathrm{Q}_1}\)

Refrigerator : Refrigerator is a heat pump which is the re verse of a heat engine.

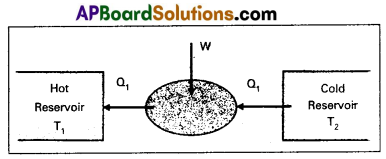
In refrigerator the working substance extracts (Q₂) an amount of heat from sink of lower temperature and a external work W is done on the working substance and finally amount of heat (Q₁) is transferred to source.
The coefficient fo performance (α) of a refrigerator is given by
α = \(\frac{Q_2}{W}=\frac{Q_2}{Q_1-Q_2}\) heat released Q₁ = Q₂ + W
For heat engine η can never exceed 1. For refrigerator α can be greater than 1. Therefore working of refrigerator is opposite to that of heat engine.