TS Inter 1st Year Maths 1B Question Paper March 2019

Access to a variety of TS Inter 1st Year Maths 1B Model Papers and TS Inter 1st Year Maths 1B Question Paper March 2019 allows students to familiarize themselves with different question patterns.

TS Inter 1st Year Maths 1B Question Paper March 2019

Time : 3 Hours
Max. Marks : 75

Section – A
(10 × 2 = 20)

I. Very Short Answer Type Questions.

1. Answer all questions.
2. Each question carries two marks.

Time : 3 Hours
Max. Marks : 75

Note : This question paper consists of THREE sections A, B and C.

Section – A
(10 × 2 = 20 Marks)

I. Very short answer type questions :

1. Answer all the questions.
2. Each question carries two marks.

Question 1.
Compute : \(\lim _{x \rightarrow 0} \frac{a^x-1}{b^x-1}\).
Solution:

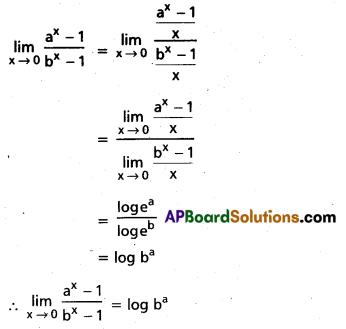
Question 2.
Find the value of p, if the straight lines 3x + py – 1 = 0, 7x – 3y + 3 = 0 are mutually perpendicular.
Solution:
Given straight line equations are
3x + py – 1 = 0 …….. (1)
7x – 3y + 3 = 0 …..(2)
Since (1), (2) are mutually perpendicular
∴ 3(7) + p(-3) = 0
⇒ 21 – 3p = 0
⇒ 3p = 21
⇒ p = 7

Question 3.
If f(x) = log(tan e^x), then find f(x).
Solution:
Given f(x) = log(tan e^x)
differentiating w.r.to ‘x’ on bothsides, we have

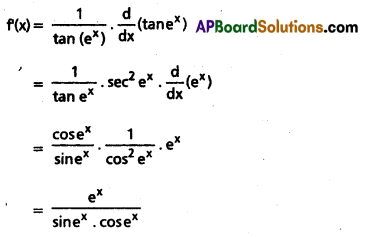
Question 4.
Find the ratio in which the xz-plane divides the line joining A(-2, 3, 4) and B(1, 2, 3).
Solution:
The ratio in which the XZ-plane divides the line joining A(-2, 3, 4) and B(1, 2, 3) is -y₁ : y₂
= 3 : 2
= 3 : 2 externally.

Question 5.
Reduce the equation of the plane x + 2y – 3z – 6 = 0 to the normal form.
Solution:
Given plane equation is x + 2y – 3z – 6 = 0

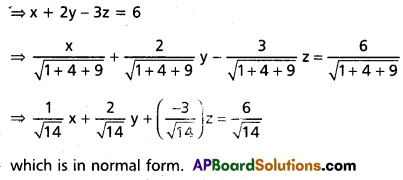
Question 6.
Evaluate: \(\lim _{x \rightarrow 0} \frac{\log _e(1+5 x)}{x}\)
Solution:

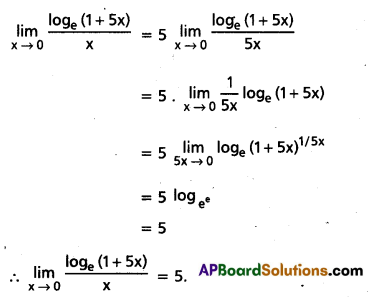
Question 7.
If f(x) = 1 + x + x² + ……… + x¹⁰⁰, then find f'(1).
Solution:
Given f(x) = 1 + x + x² + ……. + x¹⁰⁰
f'(x) = 0 + 1 + 2x + …….. + 100 x⁹⁹
f'(1) = 0 + 1 + 2(1) + ……. + 100(1)⁹⁹
f'(1) = 1 + 2 + 3 + …… + 100
= Σ100
= \(\frac{100(100+1)}{2}\)
= 50(101)
∴ f'(1) = 5050.

Question 8.
Find the angle which the straight line y = \(\sqrt{3}\)x – 4 makes with the y-axis.
Solution:
Given straight line is y = \(\sqrt{3}\)x – 4
m = \(\sqrt{3}\) ⇒ tan θ = \(\sqrt{3}\)
⇒ θ = 60°
∴ The straight line y = \(\sqrt{3}\)x – 4 makes angle with y-axis is 90° – 60° = 30°.

Question 9.
Verify Rolle’s theorem for the function y = f(x) = x² + 4 in [-3, 3],
Solution:
Given f(x) = x² + 4
Since f is a second degree polynomial
∴ f is continuous on [-3, 3] and f is derivable on (-3, 3)
Also f(-3) = (-3)² + 4
= 9 + 4 = 13
f(3) = 3² + 4
= 9 + 4 = 13
∴ f(-3) = f(3)
∴ f satisfies all the conditions of RoHe’s theorem.
∴ There exists c ∈ (-3, 3) such that f(c) = 0
f(x) = x² + 4
⇒ f(x) = 2x
⇒ f'(c) = 2c
⇒ 0 = 2c
⇒ c = 0 ∈ (-3, 3)
Hence Rolle’s theorem is verified.

Question 10.
Find ∆y and dy for the function y = cos x at x = 60° with ∆x = i.
(cos 6i = 0.4848, i = 0.0174 radians)
Solution:
Given y = f(x) cos x, x = 60°, ∆x = 1 °
∆y = f(x + ∆x) – f(x)
= f(60° + 1°) – f(60°)
= f(61 °) – f(60°)
= cos 61°- cos 60°
= 0.4848 – 0.5000
= -0.0152
dy = f'(x) ∆x
= -sin x. ∆x
= -sin 60°. (1°)
= \(\frac{\sqrt{3}}{2}\) .(0.0174)
= -(0.866) (0.0174)
= – 0.01506.

Section – B

II. Short answer type questions :

1. Attempt Any Five questions.
2. Each question carries Four marks.

Question 11.
Check the continuity of the following function at ‘2’:

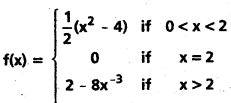
Solution:

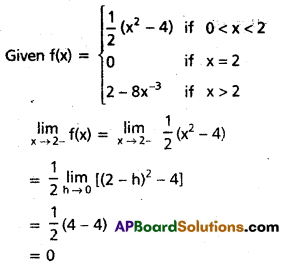
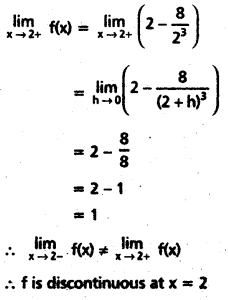
Question 12.
A(1, 2), B(2, -3) and C(-2, 3) are three points. If a point P moves such that PA² + PB² = 2PC², then show that the equation to the locus of P is 7x – 7y + 4 = 0.
Solution:
Given A = (1, 2)
B = (2, -3)
C = (-2, 3)
Let P (x₁, y₁) be a point on locus.
Given geometric condition is PA² + PB² = 2 PC²

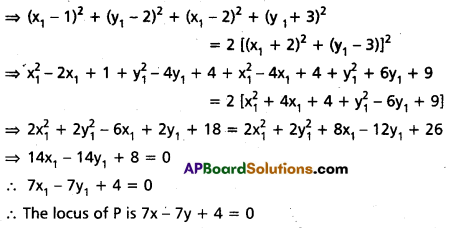
Question 13.
A straight line through Q(\(\sqrt{3}\), 2) makes an angle of \(\frac{\pi}{6}\) with the positive direction of the X-axis. If the straight line intersects the line \(\sqrt{3}\)x – 4y + 8 = 0 at P, then find the distance of PQ.
Solution:
Given straight line equation is \(\sqrt{3}\)x – 4y + 8 = 0 …. (1)
Slope of \(\overline{\mathrm{PQ}}\) = tan \(\frac{\pi}{6}\) = tan 30° = \(\frac{1}{\sqrt{3}}\)
Also Q = (\(\sqrt{3}\), 2)
Equation of \(\overline{P Q}\) is

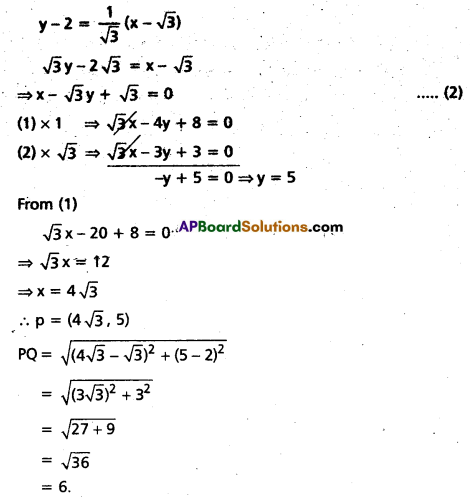
Question 14.
When the axes are rotated through an angle a, find the transformed equation of x cos α + y sin α = p.
Solution:
The given equation x cos α + y sin α = P
The axes are rotated through an angle ‘α’
x = X cos α – Y sin α
y = X sin α + Y cos α
∴ given equation transformed to
(X cos α – Y sin α) cos α + (X sin α + Y cos α) sin α = P
⇒ X cos² α – Y sin α cos α + X sin² α + Y cos α sin α = P
⇒ X (cos² α + sin² α) = p
⇒ X = P.

Question 15.
Show that the tangent at any point θ on the curve x = c sec θ, y = c tan θ is y sin θ = x – c cos θ.
Solution:
Given x = c sec θ
\(\frac{d x}{d \theta}\) = c sec θ tan θ
y = c tan θ
\(\frac{d y}{d \theta}\) = c sec²θ
slope of the tangent at any point ’θ‘ is

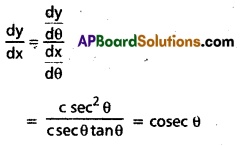
∴ The equation of the tangent is
y – c tan θ = cosec θ (x – c sec θ)

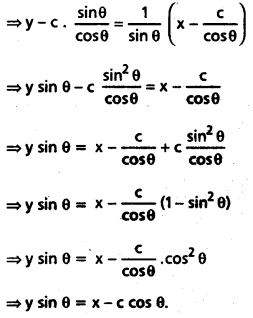
Question 16.
Find the derivative of cos²x from the first principle.
Solution:
Let f(x) = cos² x
f(x+ h) = cos²(x + h)
By first principle

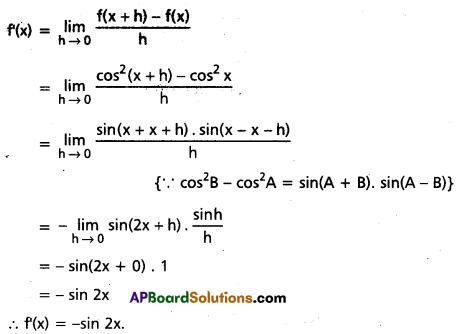
Question 17.
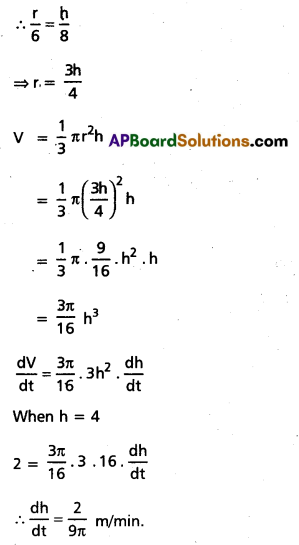
A container is in the shape of an inverted cone has height 8 m and radius 6 m at the top. If it is filled with water at the rate of 2 m³/minute, how fast is the height of water changing when the level is 4 m.
Solution:
Let OC = h and CD = r

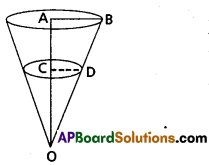
Given AB = 6, OA = 8 and \(\frac{d V}{d t}\) = 2m³/min
From fig ∆OAB, ∆OCD are similar triangles

Section – C
(5 × 7 = 35 Marks)

III. Long answer type questions :

1. Attempt Any Five questions.
2. Each question carries Seven marks.

Question 18.
Find the orthocentre of the triangle whose vertices are (5, -2), (-1, 2) and (1, 4).
Solution:
Let A = (5, -2)
B = (-1, 2)
C = (1, 4)

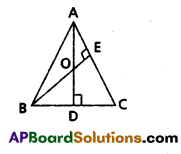
Slope of \(\overline{\mathrm{BC}}\) = \(\frac{4-2}{1-(-1)}\) = \(\frac{2}{2}\) = 1
Since \(\overline{\mathrm{AD}}\) ⊥ \(\overline{\mathrm{BC}}\)
∴ Slope of \(\overline{\mathrm{AD}}\) = \(\frac{-1}{1}\) = -1
Equation of \(\overline{\mathrm{AD}}\) is
y – (-2) = -1 (x – 5)
⇒ y + 2 = -x + 5
⇒ x + y – 3 = 0 ……. (1)
Slope of \(\overline{\mathrm{AC}}\) = \(\frac{4+2}{1-5}\)
= \(\frac{6}{-4}\) = \(\frac{-3}{2}\)
Since \(\overline{\mathrm{BE}}\) ⊥ \(\overline{\mathrm{AC}}\)
∴ Slope of \(\overline{\mathrm{BE}}\) = \(\frac{-1}{-3 / 2}\) = \(\frac{2}{3}\)
∴ Equation of \(\overline{\mathrm{BE}}\)
⇒ y – 2 = \(\frac{2}{3}\)(x + 1)
⇒ 3y – 6 = 2x + 2
⇒ 2x – 3y + 8 = 0 ……… (2)
Solving (1) and (2)

Question 19.
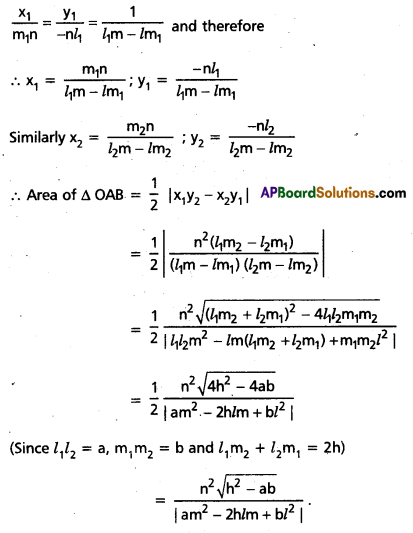
Show that the area of the triangle formed by the lines ax² + 2hxy + by² = 0 and lx + my + n = 0 is \(\left|\frac{\mathrm{n}^2 \sqrt{\mathrm{h}^2-\mathrm{ab}}}{\mathrm{am^{2 } – 2 \mathrm { h } l \mathrm { m } + \mathrm { b } l ^ { 2 }}}\right|\).
Solution:
Let \(\overleftrightarrow{\mathrm{OA}}\) and \(\overleftrightarrow{\mathrm{OB}}\) be the pair of straight lines represented by the equation

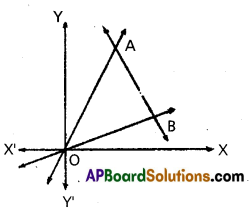
ax² + 2hxy + by² = 0 (see figure)
and \(\stackrel{\leftrightarrow}{\mathrm{AB}}\) be the line lx + my + n = 0
Let ax² + 2hxy + by² ≡ (l₁x + m₁y)(l₂x + m₂y),
and \(\overleftrightarrow{O A}\) and \(\overleftrightarrow{O B}\) be the lines.
l₁x + m₁y = 0 and
l₂x + m₂y = 0 respectively.
Let A = (x₁, y₁) and B = (x₂, y₂).
Then l₁x₁ + m₁y₁ = 0 and lx₁ + my₁ + n = 0.
So, by the rule of cross—multiphcation, we obtain

Question 20.
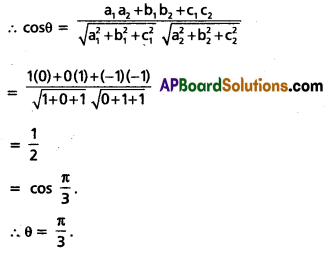
Find the angle between the lines whose direction cosines satisfy the equations :
l + m + n = 0, l² + m² – n² = 0.
Solution:
Given l + m + n = 0 …… (1)
l² + m² – n² = 0 ……. (2)
From (1) l = – m – n …… (3)
Substituting in (2)
(- m – n)² + (m² – n²) = 0.
⇒ (m + n)² + (m + n) (m – n) = 0.
⇒ (m + n) [m + n + m – n] = 0
⇒ 2m (m + n) = 0
⇒ m = 0, m + n = 0
When m = 0
From (3), l = -n
∴ \(\frac{l}{l}\) = \(\frac{m}{0}\) = \(\frac{n}{-l}\)
When m = – n
From (3) l = -(-n) – n
= n – n
= 0.
∴ \(\frac{l}{0}\) = \(\frac{m}{l}\) = \(\frac{n}{-l}\)
∴ The d.rs of the two lines are (1, 0, -1) and (0, 1, -1) let ‘θ’ be the angle between the lines.

Question 21.
If x^{log y} = log x, then show that: \(\frac{\mathrm{dy}}{\mathrm{dx}}\) = \(\frac{y}{x}\left(\frac{1-\log x \log y}{(\log x)^2}\right)\)
Solution:
Given x^{log y} = log x
Taking logarithms on bothsides, we have
log x^{log y} = log(log x)
⇒ (log y) (log x) = log (log x)
differentiating w.r. to ‘x’ on bothsides, we have

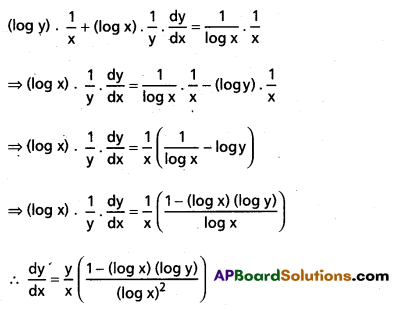
Question 22.
If the tangent at any point on the curve x^2/3 + y^2/3 = a^2/3 intersects the co-ordinate axes in A and B, then show that the length AB is a constant.
Solution:
Equation of the curve is x^2/3 + y^2/3 = a^2/3

Differentiate w.r.to x

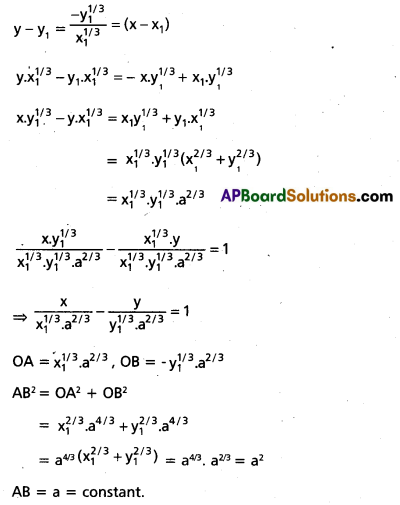
Question 23.
Find the values of k, if the lines joining the origin to the points of intersection of the curve 2x² – 2xy + 3y² + 2x – y – 1 = 0 and the line x + 2y = k are mutually perpendicular.
Solution:
The given circle equation
S ≡ 2x² + 2xy + 3y² + 2x – y – 1 = 0 …… (1)

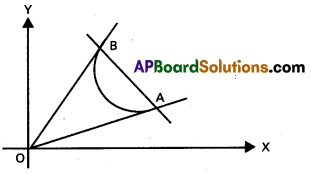
AB equation x + 2y = k
\(\frac{x+2 y}{k}\) = 1 ……… (2)
Homogenising (1) with the help of (2) combined equation of OA, OB is
2x² – 2xy + 3y² + 2x.1 – y.1 – 1² = 0
2x² – 2xy + 3y² + 2x\(\frac{(x+2 y)}{k}\) – y\(\frac{(x+2 y)}{k}\) – \(\frac{(x+2 y)^2}{k}\) = 0
Multiplaing with k²
2k²x² – 2k²xy + 3k²y² + 2kx (x + 2y) – ky (x + 2y) – (x + 2y)² = 0
2k²x² – 2k²xy + 3k²y² + 2kx² + 4kxy – kxy – 2ky² – x² – 4xy – 4y² = 0
(2k² + 2k – 1) x² + (-2k² + 3k – 4) xy + (3k² – 2k – 4) y² = 0
since OA, OB are perpendicular.
x² + y² = 0
2k² + 2k – 1 + 3k² – 2k – 4 = 0
5k² = 5 ⇒ k² = 1
∴ k = ± 1.

Question 24.
Find the maximum area of the rectangle that can be formed with fixed perimeter 20.
Solution:
Let x and y denote the length and breadth of a rectangle.
Given 2(x + y) = 20
⇒ x + y = 10 ⇒ y = 10 – x ……… (1)
Let A be the area of the rectangle.
∴ A = xy
= x(10 – x)
= 10x – x²
\(\frac{\mathrm{dA}}{\mathrm{dx}}\) = 10 – 2x
\(\frac{\mathrm{dA}}{\mathrm{dx}}\) = 0 ⇒ 10 – 2x = 0 dx
⇒ 2x = 10.
⇒ x = 5
\(\frac{\mathrm{d}^2 \dot{A}}{\mathrm{dx}^2}\) = 0 – 2
= -2 < 0
\(\left(\frac{d^2 A}{d x^2}\right)_{x=5}\) = -2 < 0
Rectangle area is maximum at x = 5
∴ y = 10 – x
= 10 – 5
= 5
Maximum are A = xy
= 5(5) = 25