AP Inter 2nd Year Maths 2A Question Paper March 2019

Thoroughly analyzing AP Inter 2nd Year Maths 2A Model Papers and AP Inter 2nd Year Maths 2A Question Paper March 2019 helps students identify their strengths and weaknesses.

AP Inter 2nd Year Maths 2A Question Paper March 2019

Time: 3 Hours
Maximum Marks: 75

Note: This question paper consists of three sections A, B, and C.

Section – A
(10 × 2 = 20 Marks)

I. Very Short Answer type Questions.

• Attempt all the questions.
• Each question carries two marks.

Question 1.
If (√3 + i)¹⁰⁰ = 2⁹⁹ (a + ib), then show that a² + b² = 4.
Solution:

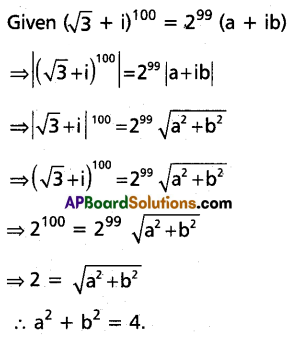
Question 2.
If z = 2 – 3i, then show that z² – 4z + 13 = 0.
Solution:
Given z = 2 – 3i
⇒ z – 2 = -3i
⇒ (z – 2)² = (-3i)²
⇒ z² – 4z + 4 = 9i²
⇒ z² – 4z + 4 = -9
⇒ z² – 4z + 13 = 0

Question 3.
If 1, w, w² are the cube roots of unity, then find the value of (1 – w + w²)⁵ + (1 – w + w²)⁵.
Solution:
Since 1, w, w² are the cube roots of unity
∴ 1 + w + w² = 0 and w³ = 1
(1 – w + w²)5 + (1 + w – w²)⁵ = (-w – w)⁵ + (-w² – w²)⁵
= (-2w)⁵ + (-2w²)⁵
= -32w⁵ – 32w¹⁰
= -32w² – 32w
= -32(w² + w)
= -32(-1)
= 32
∴ (1 – w + w²)⁵ + (1 + w – w²)⁵ = 32

Question 4.
Find the values of m, for which the equation x² – 15 – m(2x – 8) = 0 have equal roots.
Solution:
Given equation x² – 15 – m(2x – 8) = 0
⇒ x² – 15 – 2mx + 8m = 0
⇒ x² – 2mx + (8m – 15) = 0 ………(1)
Since (1) have equal roots.
∴ (-2m)² – 4 (1) (8m – 15) = 0
⇒ 4m² – 4(8m – 15) = 0
⇒ m² – (8m – 15) = 0
⇒ m² – 8m + 15 = 0
⇒ m² – 3m – 5m + 15 = 0
⇒ m(m – 3) – 5(m – 3) = 0
⇒ (m – 3) (m – 5) = 0
⇒ m = 3, 5

Question 5.
If the product of the roots of 4x³ + 16x² – 9x – a = 0 is 9, then find a.
Solution:
Given equation 4x³ + 16x² – 9x – a = 0
Given product of the roots = 9
⇒ \(\frac{-(-a)}{4}\) = 9
⇒ a = 36

Question 6.
Find the number of ways of arranging the letters of the word INTERMEDIATE.
Solution:
The number of ways of arranging the letters of the word INTERMEDIATE = \(\frac{12 !}{2 ! 2 ! 3 !}\) {∴ 2I’s, 2T’s, 3E’s}

Question 7.
If ⁿC₅ = ⁿC₆, then find ¹³C_n.
Solution:
Given ⁿC₅ = ⁿC₆
∴ n = 5 + 6 = 11
¹³C_n = ¹³C₁₁
= \(\frac{13.12}{2 !}\)
= 13 × 6
= 78
∴ ¹³C_n = 78

Question 8.
Find the 6th term in the expansion of \(\left(\frac{2 x}{3}+\frac{3 y}{2}\right)^9\).
Solution:
T₆ = T₅₊₁

∴ 6th term in the expansion of \(\left(\frac{2 x}{3}+\frac{3 y}{2}\right)^9\) is \(9_{c_5} \frac{3}{2} \cdot x^4 \cdot y^5\)

Question 9.
Find the mean deviation about the median for the following data:
4, 6, 9, 3, 10, 13, 2
Solution:
Given ungrouped data are 4, 6, 9, 3, 10, 13, 2.
Expressing the data in ascending order of magnitude, we have 2, 3, 4, 6, 9, 10, 13.
∴ Median = 6
The absolute values are |6 – 2|, |6 – 3|, |6 – 4|, |6 – 6|, |6 – 9|, |6 – 10|, |6 – 13| = 4, 3, 2, 0, 3, 4,7
∴ Mean deviation from the median = \(\frac{4+3+2+0+3+4+7}{7}\)
= \(\frac{23}{7}\)
= 3.285

Question 10.
The mean and variance of a binomial distribution are 4 and 3 respectively, fix the distribution and find P(X ≥ 1).
Solution:
Let x follow a binomial distribution with parameters n and p.
Given mean = 4 ⇒ np = 4
Variance = 3 ⇒ npq = 3
\(\frac{n p q}{n p}=\frac{3}{4}\)
⇒ q = \(\frac{3}{4}\)
∴ p = 1 – q
= 1 – \(\frac{3}{4}\)
= \(\frac{1}{4}\)
∴ np = 4
⇒ n(\(\frac{1}{4}\)) = 4
⇒ n = 16
∴ n = 16 and p = \(\frac{1}{4}\)
p(x ≥ 1) = 1 – p(x = 0)
= 1 – \(16_{c_0}\left(\frac{1}{4}\right)^0\left(\frac{3}{4}\right)^{16-0}\)
= 1 – \(\left(\frac{3}{4}\right)^{16}\)

Section – B
(5 × 4 = 20 Marks)

II. Short Answer Type Questions.

• Attempt any five questions.
• Each question carries four marks.

Question 11.
If x + iy = \(\frac{1}{1+\cos \theta+i \sin \theta}\), then show that 4x² – 1 = 0.
Solution:
Given x + iy = \(\frac{1}{1+\cos \theta+i \sin \theta}\)

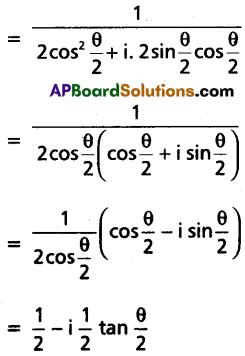
Now, equating real points on both sides, we have
x = \(\frac{1}{2}\)
⇒ x² = \(\frac{1}{4}\)
⇒ 4x² = 1
⇒ 4x² – 1 = 0

Question 12.
If x is real, prove that \(\frac{x}{x^2-5 x+9}\) lies between \(\frac{-1}{11}\) and 1.
Solution:
Let y = \(\frac{x}{x^2-5 x+9}\)
⇒ yx² – 5xy + 9y = x
⇒ yx² + (-5y – 1)x + 9y = 0
x ∈ R ⇒ (-5y – 1)² – 4 . y . 9y ≥ 0
⇒ 25y² + 10y + 1 – 36y² ≥ 0
⇒ -11y² + 10y + 1 ≥ 0
⇒ 11y² – 11y + y – 1 ≥ 0
⇒ 11y – (y – 1) + 1(y – 1) ≥ 0
⇒ (11y + 1)(y – 1) ≥ 0
⇒ \(\frac{-1}{11}\) ≤ y ≤ 1
∴ \(\frac{x}{x^2-5 x+9}\) lies between \(\frac{-1}{11}\) and 1.

Question 13.
If the letters of the word MASTER are permuted in all possible ways and the words thus formed are arranged in the dictionary order, then find the rank of the word MASTER.
Solution:
The alphabetical order of the letters of the word REMAST is A, E, M, R, S, T
The no.of words beginning with A is 5! = 120
The no.of words begin with E is 5! = 120
The no.of words begin with MAE is 3! = 6
The no.of words begin with MAR is 3! = 6
The no.of words begin with MASE is 2! = 2
The no.of words beginning with MASR is 2! = 2
The next word is MASTER = 1
∴ Rank of the word MASTER = 120 + 120 + 6 + 6 + 2 + 2 + 1 = 257

Question 14.
Simplify: ³⁴C₅ + \(\sum_{r=0}^4(38-r) C_4\).
Solution:

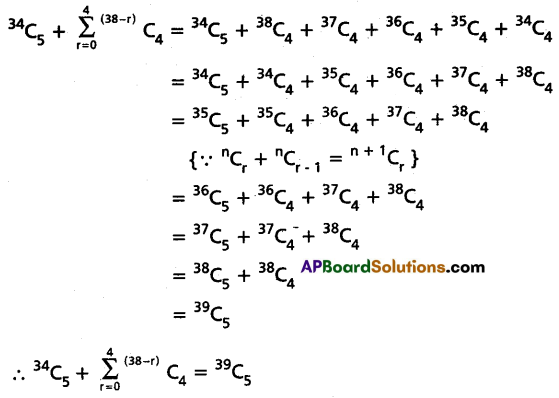
Question 15.
Resolve \(\frac{x^3}{(x-1)(x+2)}\) into partial fractions.
Solution:
(x – 1) (x + 2) = x² + 2x – x – 2 = x² + x – 2

∴ \(\frac{x^3}{(x-1)(x+2)}=x-1+\frac{3 x-2}{(x-1)(x+2)}\)
Let \(\frac{3 x-2}{(x-1)(x+2)}=\frac{A}{x-1}+\frac{B}{x-2}\)
⇒ \(\frac{3 x-2}{(x-1)(x+2)}=\frac{A(x+2)+B(x-1)}{(x-1)(x+2)}\)
⇒ 3x – 2 = A(x + 2) + B(x – 1)
put x = 1
3 – 2 = A(1 + 2)
⇒ 1 = 3A
⇒ A = \(\frac{1}{3}\)
put x = -2
3(-2) – 2 = B(-2 – 1)
⇒ -8 = -3B
⇒ B = \(\frac{8}{3}\)
∴ \(\frac{3 x-2}{(x-1)(x+2)}=\frac{1 / 3}{x-1}+\frac{8 / 3}{x+2}\)
∴ \(\frac{3 x}{(x-1)(x+2)}=x-1+\frac{1}{3(x-1)}+\frac{8}{3(x+2)}\)

Question 16.
A, B, C are three horses in a race. The probability of A winning the race is twice that of B, and the probability of B is twice that of C. What are the probabilities of A, B, and C to win the race?
Solution:
Let A, B, and C be the events where the horses A, B, and C win the race.
Given P(A) = 2P(B), P(B) = 2P(C)
∴ P(A) = 2P(B)
= 2[2P(C)]
= 4P(C)
Since the horses A, B and C run the race
A ∪ B ∪ C = S and A, B, C are mutually disjoint.
P(A ∪ B ∪ C) = P(A) + P(B) + P(C)
⇒ P(S) = 4P(C) + 2P(C) + P(C)
⇒ 1 = 7 P(C)
⇒ P(C) = \(\frac{1}{7}\)
P(A) = 4P(C) = 4 × \(\frac{1}{7}\) = \(\frac{4}{7}\)
P(B) = 2P(C) = 2 × \(\frac{1}{7}\) = \(\frac{2}{7}\)
∴ P(A) = \(\frac{4}{7}\), P(B) = \(\frac{2}{7}\) and P(C) = \(\frac{1}{7}\)

Question 17.
A speaks truth in 75% of the cases and B in 80% of cases. What is the probability that their statements about an incident do not match?
Solution:
Let E₁ and E₂ be the events that A and B respectively speak the truth about an incident.
∴ P(E₁) = \(\frac{75}{100}=\frac{3}{4}\)
⇒ P(\(\mathrm{E}_1^{\mathrm{c}}\)) = 1 – \(\frac{3}{4}\) = \(\frac{1}{4}\)
∴ P(E₂) = \(\frac{80}{100}=\frac{4}{5}\)
⇒ P(\(\mathrm{E}_2^{\mathrm{c}}\)) = 1 – \(\frac{4}{5}\) = \(\frac{1}{5}\)
If E is the event that their statements do not match about the incident, then this happens in two mutually exclusive ways.
(i) A speaks the truth and B tells a lie.
(ii) A tells lie and B speaks truth.
∴ P(E) = \(P\left(E_1 \cap E_2^c\right)+P\left(E_1^c \cap E_2\right)\)
= \(P\left(E_1\right) \cdot P\left(E_2^c\right)+\left(E_1^c\right) \cdot P\left(E_2\right)\)
= \(\left(\frac{3}{4}\right)\left(\frac{1}{5}\right)+\left(\frac{1}{4}\right)\left(\frac{4}{5}\right)\)
= \(\frac{3}{4}+\frac{4}{20}\)
= \(\frac{7}{20}\)

Section – C
(5 × 7 = 35 Marks)

III. Long Answer Type Questions.

• Attempt any five questions.
• Each question carries seven marks.

Question 18.
If α, β are the roots of the equation x² – 2x + 4 = 0, then for any n ∈ N, show that \(\alpha^n+\beta^n=2^{n+1} \cdot \cos \left(\frac{n \pi}{3}\right)\).
Solution:

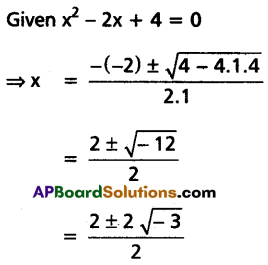
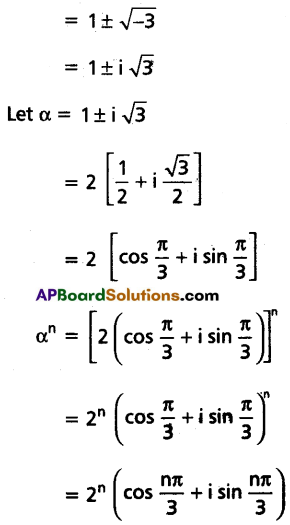
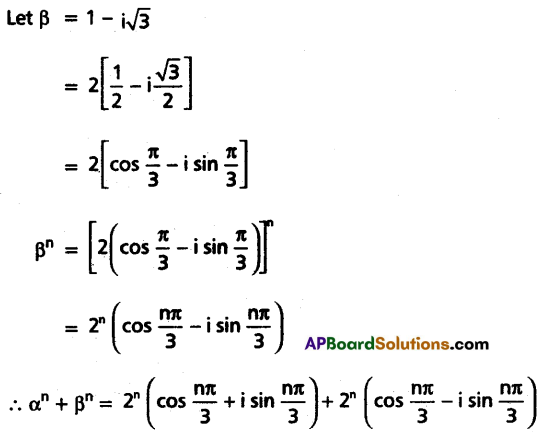
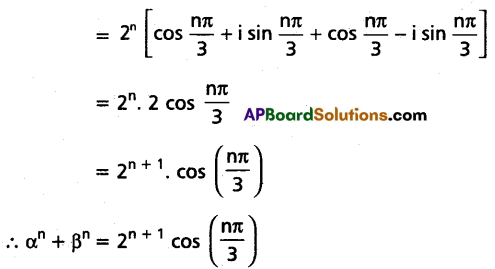
Question 19.
Solve 18x³ + 81x² + 121x + 60 = 0, given that one root is equal to half the sum of the remaining roots.
Solution:
Given 18x³ + 81x² + 121x + 60 = 0 ………(1)
Since one root is equal to half the sum of the remaining roots.
∴ The roots of (1) are in A. P.
Let the roots be a – d, a, a + d
∴ (a – d) + a + (a + d) = \(\frac{-81}{18}\)
⇒ 3a = \(\frac{-9}{2}\)
⇒ a = \(\frac{-3}{2}\)
(a – d) (a) (a + d) = \(\frac{-60}{18}\)
⇒ a(a² – d²) = \(\frac{-10}{3}\)
⇒ \(\frac{-3}{2}\left(\frac{9}{4}-d^2\right)=\frac{-10}{3}\)
⇒ \(\frac{9}{4}-d^2=\frac{20}{9}\)

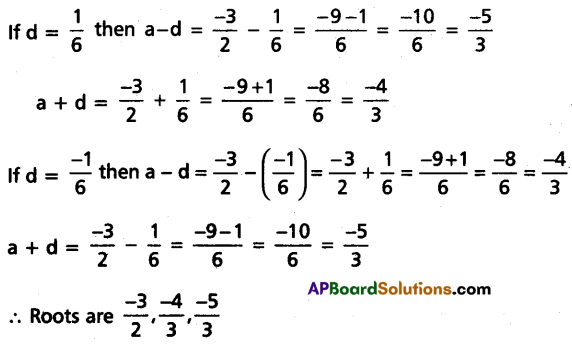
Question 20.
If the co-efficient of x¹⁰ in the expansion of \(\left(a x^2+\frac{1}{b x}\right)^{11}\) is equal to the coefficient of x^-10 in the expansion of \(\left(a x-\frac{1}{b x^2}\right)^{11}\), then find the relation between a and b where a and b are real numbers.
Solution:
The general term in the expansion of \(\left(a x^2+\frac{1}{b x}\right)^{11}\) is
\(T_{r+1}={ }^{11} C_r\left(a x^2\right)^{11-r}\left(\frac{1}{b x}\right)^r\)
= \({ }^{11} C_r a^{11-r} x^{22-2 r} \frac{1}{b^r} \cdot \frac{1}{x^r}\)
= \({ }^{11} C_r a^{11-r} \frac{1}{b^r} \cdot x^{22-3 r}\)
For the co-efficient of x¹⁰
22 – 3r = 10
⇒ 3r = 12
⇒ r = 4
∴ The co-efficient of x¹⁰ in the expansion of \(\left(a x^2+\frac{1}{b x}\right)^{11}\) is \({ }^{11} C_4 a^7 \cdot \frac{1}{b^4}\) ………(1)
The general term in the expansion of \(\left(a x-\frac{1}{b x^2}\right)^{11}\) is

For the co-efficient of x¹⁰
11 – 3r = -10
⇒ 3r = 21
⇒ r = 7
∴ The co-efficient of x¹⁰ in the expansion of \(\left(a x-\frac{1}{b x^2}\right)^{11}\) = \((-1)^{711} C_r a^{11-7} \cdot \frac{1}{b^7}\) = \({ }^{11} C_7 a^4 \cdot \frac{1}{b^7}\) …….(2)
From the hypothesis (1) and (2) are equal.
∴ \({ }^{11} C_4 a^7 \cdot \frac{1}{b^4}=-{ }^{11} C_7 a^4 \cdot \frac{1}{b^7}\)
⇒ a³ = \(\frac{-1}{b^3}\)
⇒ a³b³ = -1
⇒ (ab)³ = -1
⇒ ab = -1

Question 21.
If x = \(\frac{1.3}{3.6}+\frac{1.3 .5}{3.6 .9}+\frac{1.3 .5 .7}{3 \cdot 6 \cdot 9.12}\) + ………. ∞, then prove that 9x² + 24x = 11.
Solution:

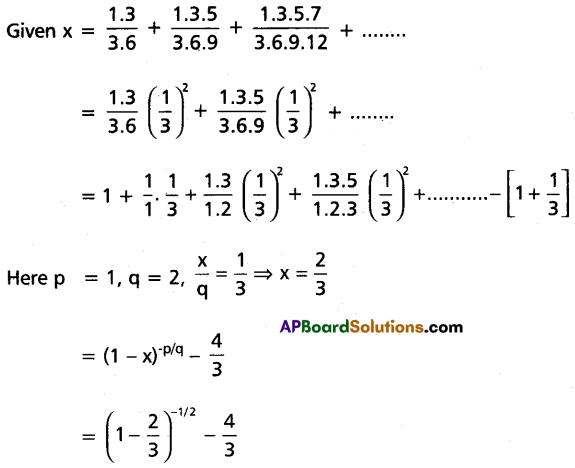
= \(\left(\frac{1}{3}\right)^{-1 / 2}-\frac{4}{3}\)
= \(\sqrt{3}-\frac{4}{3}\)
⇒ 3x + 4 = 3√3
Squaring on both sides
⇒ (3x + 4)² = (3√3)²
⇒ 9x² + 24x + 16 = 27
⇒ 9x² + 24x = 11

Question 22.
Calculate the variance and standard deviation for the following discrete frequency distribution:

xi	4	8	11	17	20	24	32
fi	3	5	9	5	4	3	1

Solution:
Construct the following table.

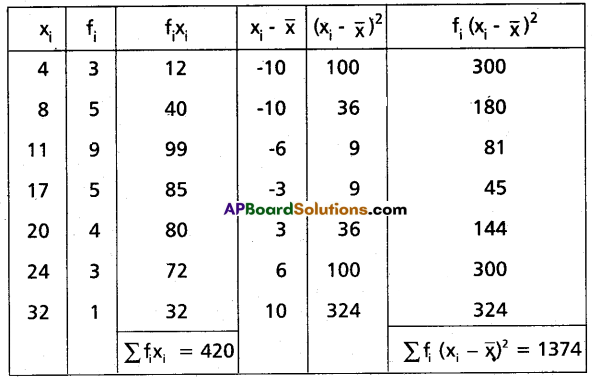
Here N = Σf_i = 30
\(\bar{x}=\frac{\sum f_i x_i}{N}=\frac{430}{30}\)
Variance (σ2) = \(\frac{1}{N} \sum f_i\left(x_i-\bar{x}\right)^2\)
= \(\frac{1374}{30}\)
= 45.8
Standard deviation = \(\sqrt{45.8}\) = 6.77

Question 23.
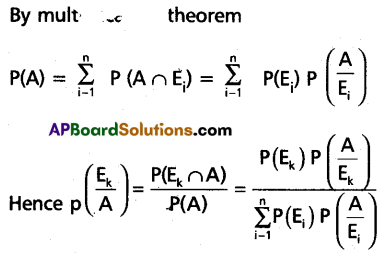
State and prove Baye’s Theorem.
Solution:
Statement: Let E₁, E₂,………., E_n, are mutually exclusive and exhaustive events or a random experiment with P(E_i) ≠ 0 for i = 1, 2, 3,……, n. Then for any event A or the random experiment
with P(A) ≠ 0
\(P\left(\frac{E_k}{A}\right)=\frac{P\left(E_k\right) P\left(\frac{A}{E_k}\right)}{\sum_{i=1}^n P\left(E_i\right) P\left(\frac{A}{E_i}\right)}\), k = 1, 2, …….., n
Proof: Given that P(E₁) > 0 for i = 1, 2,……, n
By hypothesis i ≠ j
E_i ∩ K_j = φ and E₁ ∪ E₂ ∪ ……. ∪ E_n = S
A = A ∩ S

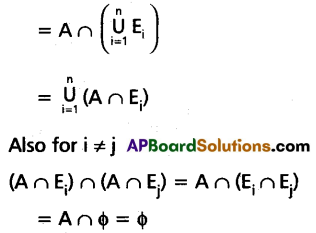
Question 24.
The probability distribution of a random variable X is given below:

X = xi	1	2	3	4	5
P(X = xi)	k	2k	3k	4k	5k

Find the value of k and the mean and variance of X.
Solution:
We have \(\sum_{i=1}^5 P\left(X=x_i\right)\) = 1
⇒ k + 2k + 3k + 4k + 5k = 1
⇒ k = \(\frac{1}{15}\)
Mean µ of x = \(\sum_{i=1}^5 r \cdot P\left(X=x_i\right)\)
= \(\sum_{i=1}^5 r(r k)\)
= 1.(k) + 2.(2k) + 3.(3k) + 4.(4k) + 5.(5k)
= 55k
= 55 × \(\frac{1}{15}\)
= \(\frac{11}{3}\)
Variance (σ²) = (1)² . k + (2)² . 2k + (3)² . 3k + (4)² . 4k + (5)² . 5k – µ²
= k + 8k + 27k + 64k + 125k – \(\left(\frac{11}{3}\right)^2\)
= 225k – \(\frac{121}{9}\)
= \(225 \times \frac{1}{15}-\frac{121}{9}\)
= \(\frac{135-121}{9}\)
= \(\frac{14}{9}\)