AP Inter 1st Year Maths 1A Question Paper March 2020

Thoroughly analyzing AP Inter 1st Year Maths 1A Model Papers and AP Inter 1st Year Maths 1A Question Paper March 2020 helps students identify their strengths and weaknesses.

AP Inter 1st Year Maths 1A Question Paper March 2020

Time: 3 Hours
Maximum Marks: 75

Note: This question paper consists of three sections – A, B, and C.

Section – A
(10 × 2 = 20 Marks)

I. Very Short Answer Type Questions.

• Answer all the questions.
• Each question carries two marks.

Question 1.
If f(x) = 2x – 1, g(x) = \(\frac{x+1}{2}\) ∀ x ∈ R then find (gof) (x).
Solution:
f(x) = 2x – 1, g(x) = \(\frac{x+1}{2}\) ∀ x ∈ R
(gof) (x) = g(f(x))
= g(2x – 1), {∵ f(x) = 2x – 1}
= \(\frac{(2 x-1)+1}{2}\) {∵ g(x) = \(\frac{x+1}{2}\)}
= x
∴ (g o f) (x) = x

Question 2.
If f = {(1, 2), (2, -3), (3, -1)} then find (i) 2f (ii) f².
Solution:
Given f = {(1, 2), (2, -3), (3, -1)}
(i) 2f = {(1, 2(2)), (2, 2(-3), (3, 2(-1))} = {(1, 4), (2, -6), (3, -2)}
(ii) f² = {(1, 22), (2, (-3)2), (3, (-1)2)} = {(1, 4), (2, 9), (3, 1)}

Question 3.
Find the trace of the matrix A = \(\left[\begin{array}{ccc}
0 & 3 & -5 \\
2 & -1 & 5 \\
1 & 0 & 1
\end{array}\right]\).
Solution:
Trace of the matrix A = \(\left[\begin{array}{ccc}
0 & 3 & -5 \\
2 & -1 & 5 \\
1 & 0 & 1
\end{array}\right]\) = Sum of the diagonal elements
= 1 – 1 + 1
= 1

Question 4.
Find the rank of the matrix A = \(\left[\begin{array}{lll}
0 & 1 & 2 \\
1 & 2 & 3 \\
3 & 2 & 1
\end{array}\right]\).
Solution:
A ~ \(\left[\begin{array}{lll}
0 & 1 & 2 \\
1 & 2 & 3 \\
3 & 2 & 1
\end{array}\right]\) (on interchanging R₁ and R₂)
~ \(\left[\begin{array}{ccc}
1 & 2 & 3 \\
0 & 1 & 2 \\
0 & -4 & -8
\end{array}\right]\)
R₃ → R₃ – 3R₁
~ \(\left[\begin{array}{ccc}
1 & 0 & -1 \\
0 & 1 & 2 \\
0 & 0 & 0
\end{array}\right]\)
R₁ → R₁ – 2R₂
R₃ → R₃ + 4R₂
The last matrix is singular and \(\left[\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right]\) is a non-singular submatrix of it. Hence its rank is 2.
∴ Rank (A) = 2.

Question 5.
If \(\overline{\mathrm{a}}=2 \overline{\mathrm{i}}+5 \overline{\mathrm{j}}+\overline{\mathrm{k}}\) and \(\overline{\mathrm{b}}=4 \overline{\mathrm{i}}+\mathrm{m} \overline{\mathrm{j}}+n \overline{\mathrm{k}}\) are collinear vectors then find m, n.
Solution:
Since \(\overline{\mathrm{a}}=2 \overline{\mathrm{i}}+5 \overline{\mathrm{j}}+\overline{\mathrm{k}}\) and \(\overline{\mathrm{b}}=4 \overline{\mathrm{i}}+\mathrm{m} \overline{\mathrm{j}}+n \overline{\mathrm{k}}\) are collinear.
⇒ \(\frac{2}{4}=\frac{5}{m}=\frac{1}{n}\)
⇒ \(\frac{1}{2}=\frac{5}{m}\) and \(\frac{1}{n}=\frac{1}{2}\)
⇒ m = 10 and n = 2

Question 6.
Find the vector equation of the plane passing through the points (0, 0, 0), (0, 5, 0), and (2, 0, 1).
Solution:

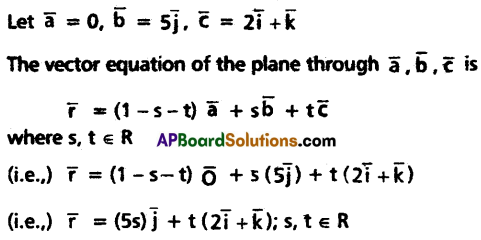
Question 7.
Find the angle between the planes \(\bar{r} \cdot(2 \bar{i}-\bar{j}+2 \bar{k})=3\) and \(\bar{r} \cdot(3 \bar{i}+6 \bar{j}+\bar{k})=4\).
Solution:

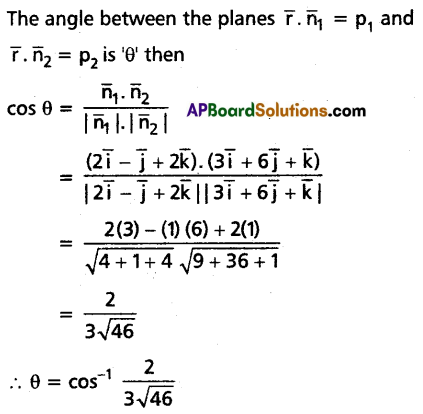
Question 8.
If tan 20° = λ then show that \(\frac{\tan 160^{\circ}-\tan 110^{\circ}}{1+\tan 160^{\circ} \tan 110^{\circ}}=\frac{1-\lambda^2}{2 \lambda}\).
Solution:

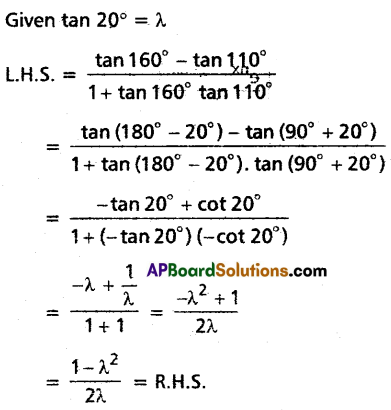
Question 9.
Find the range of 7 cos x – 24 sin x + 5.
Solution:

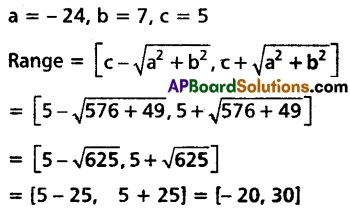
Question 10.
Prove that (cosh x – sinh x)ⁿ = cosh (nx) – sinh (nx). Such as for any n ∈ Rx.
Solution:

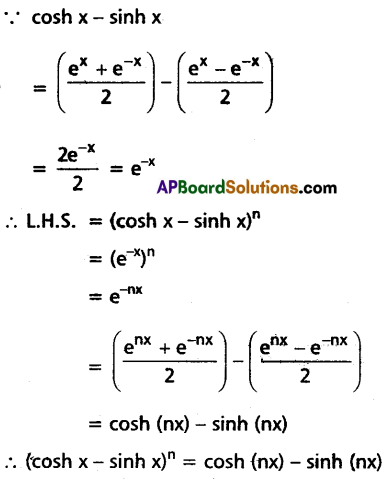
Section – B
(5 × 4 = 20 Marks)

II. Short Answer Type Questions.

• Answer any five questions.
• Each question carries four marks.

Question 11.
If A = \(\left[\begin{array}{cc}
\cos \alpha & \sin \alpha \\
-\sin \alpha & \cos \alpha
\end{array}\right]\) then show that AA’ = A’A = I.
Solution:

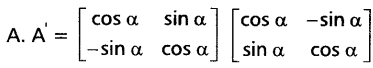
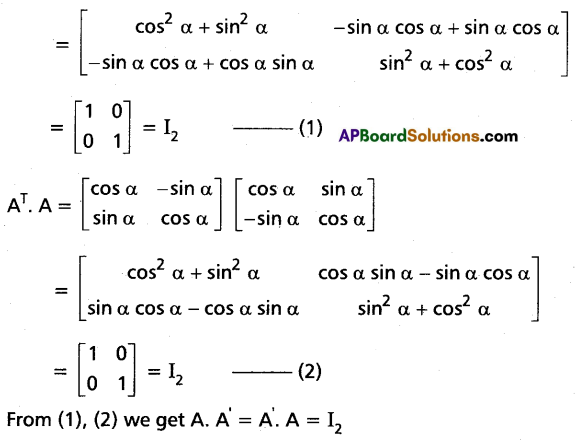
Question 12.
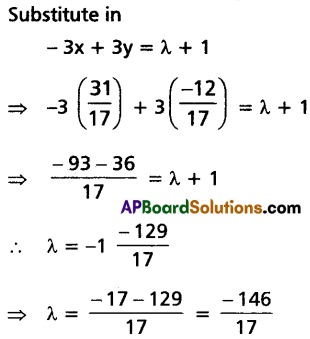
If the points whose position vectors are \(3 \bar{i}-2 \bar{j}-\bar{k}, 2 \bar{i}+3 \bar{j}-4 \bar{k},-\bar{i}+\bar{j}+2 \bar{k}\) and \(4 \bar{i}+5 \bar{j}+\lambda \bar{k}\) are coplanar then show that λ = \(\frac{-146}{17}\).
Solution:
Let ‘O’ be the origin and Let A, B, C, and D be the given points. Then

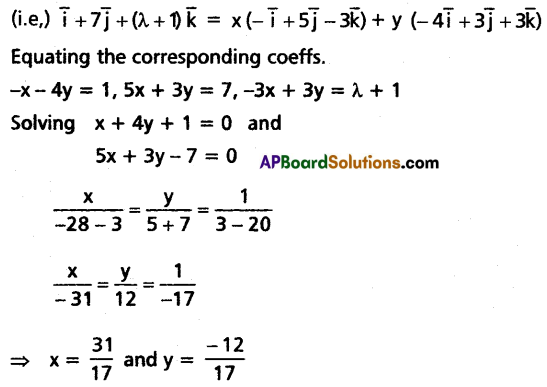
Question 13.
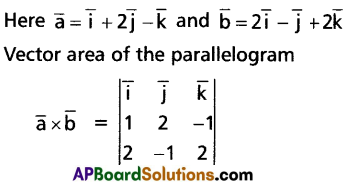
Find the vector area and area of the parallelogram having \(\overline{\mathrm{a}}=\overline{\mathrm{i}}+2 \overline{\mathrm{j}}-\overline{\mathrm{k}}\) and \(\overline{\mathrm{b}}=2 \overline{\mathrm{i}}-\overline{\mathrm{j}}+2 \overline{\mathrm{k}}\) as adjacent sides.
Solution:

Question 14.
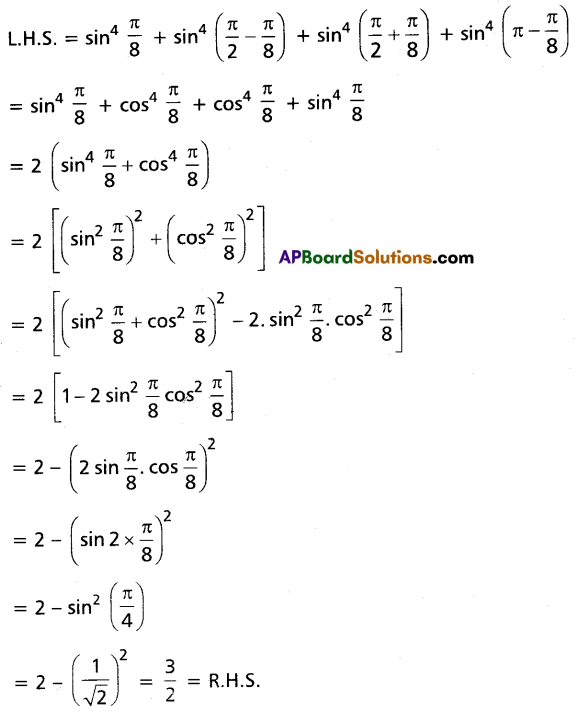
Prove that \(\sin ^4 \frac{\pi}{8}+\sin ^4 \frac{3 \pi}{8}+\sin ^4 \frac{5 \pi}{8}+\sin ^4 \frac{7 \pi}{8}=\frac{3}{2}\).
Solution:

Question 15.
Solve 7 sin²θ + 3 cos²θ = 4.
Solution:
Given that 7 sin²θ + 3 cos²θ = 4
⇒ 7 sin²θ + 3(1 – sin²θ) = 4
⇒ 4 sin²θ = 1
⇒ sin θ = ±\(\frac{1}{2}\)
∴ Principal solutions are θ = ±\(\frac{\pi}{6}\) and the general solution is given by θ = nπ ± \(\frac{\pi}{6}\), n ∈ Z.

Question 16.
Prove that \(\tan ^{-1} \frac{1}{2}+\tan ^{-1} \frac{1}{5}+\tan ^{-1} \frac{1}{8}=\frac{\pi}{4}\).
Solution:

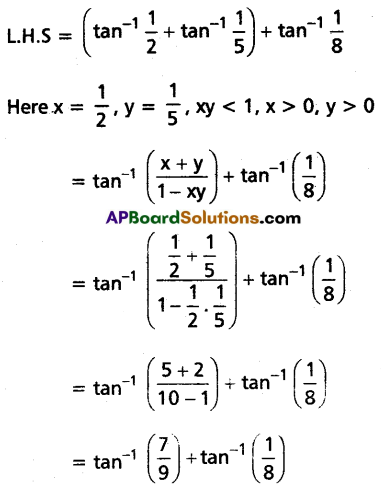
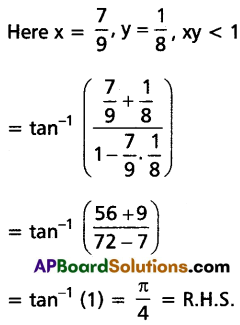
Question 17.
If cot \(\frac{A}{2}\), cot \(\frac{B}{2}\), cot \(\frac{C}{2}\) are in A.P. then prove that a, b, c are in A.P.
Solution:
cot \(\frac{A}{2}\), cot \(\frac{B}{2}\), cot \(\frac{C}{2}\) are in A.P
⇒ \(\frac{(s)(s-a)}{\Delta}, \frac{(s)(s-b)}{\Delta}, \frac{(s)(s-c)}{\Delta}\) are in A.P
⇒ s – a, s – b, s – c are in A.P
⇒ -a, -b, -c are in A.P
⇒ a, b, c are in A.P

Section – C
(5 × 7 = 35 Marks)

III. Long Answer Type Questions.

• Answer any five questions.
• Each question carries seven marks.

Question 18.
(a) If f(x) = \(\frac{x+1}{x-1}\), (x ≠ ±1) then find (fofof) (x).
(b) If f: A → B, g: B → C, h: C → D are functions then show that ho(gof) = (hog)of.
Solution:
(a) f(x) = \(\frac{x+1}{x-1}\), (x ≠ ±1)
(fofof) (x) = (fof) [f(x)]
= (fof) \(\left(\frac{x+1}{x-1}\right)\), {∵ f(x) = \(\frac{x+1}{x-1}\)}

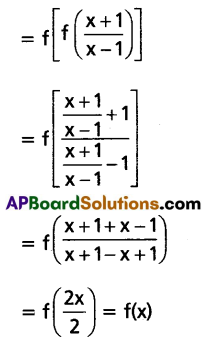
(b) f: A → B and g: B → C, gof: A → C
Now g o f: A → C and h: C → D
⇒ h o (g o f): A → D
Similarly (h o g) o f: A → D
Thus h o (g o f) and (h o g) of both exist and have the same domain A and co-domain D.
Let a be an element of A.
Now [h o (g o f)] (a) = h[(g o f)(a)]
= h[g(f(a))]
= (h o g) [f(a)]
= [(h o g) o f] (a)
∴ h o (g o f) = (h o g) o f
Thus composition of mappings is associative.

Question 19.
Show that 49ⁿ + 16n – 1 is divisible by 64 for all positive integers by using Mathematical induction.
Solution:
Let p(n) be the statement.
49ⁿ + 16n – 1 is divisible by 64
Since 49¹ + 16(1) – 1 = 64 is divisible by 64
∴ The statement is true for n = 1
Assume that the statement p(n) is true for n = k
(i.e.,) 49^k + 16k – 1 is divisible by 64
Then (49^k + 16k – 1) = 64t, for some t ∈ N ……….(1)
we show that the statement is true for n = k + 1.
(i.e.,) we show that 49^k+1 + 16(k + 1) – 1 is divisible by 64
From (1), we have 49^k + 16k – 1 = 64t
⇒ 49^k = 64t – 16k + 1
⇒ 49^k . 49 = (64t – 16k + 1) . 49
⇒ 49^k+1 + 16(k + 1) – 1 = (64t – 16k + 1)49 + 16(k + 1) – 1
⇒ 49^k+1 + 16(k + 1) -1 = 64 (49t – 12k + 1)
Here (49t – 12k + 1) is an integer.
∴ 49^k+1 + 16(k + 1) – 1 is divisible by 64.
∴ The statements true for n = k + 1
∴ By the principle of mathematical induction,
∴ p(n) is true for all n ∈ N.
(i.e.,) 49ⁿ + 16n – 1 is divisible by 64, ∀ n ∈ N.

Question 20.
Show that \(\left|\begin{array}{ccc}
a^2+2 a & 2 a+1 & 1 \\
2 a+1 & a+2 & 1 \\
3 & 3 & 1
\end{array}\right|\) = (a – 1)³.
Solution:

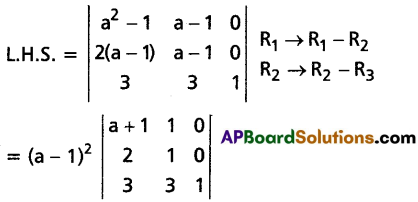
= (a – 1)² [0(6 – 3) – 0[3(a + 1) – 3)] + 1(a + 1 – 2)]
= (a – 1)² (a – 1)
= (a – 1)³
= R.H.S.

Question 21.
Solve the equations using Cramer’s Rule x + y + z = 1, 2x + 2y + 3z = 6, x + 4y + 9z = 3.
Solution:
Cramer’s rule
∆ = \(\left|\begin{array}{lll}
1 & 1 & 1 \\
2 & 2 & 3 \\
1 & 4 & 9
\end{array}\right|\)
= 1(18 – 12) – 1(18 – 3) + 1(8 – 2)
= 6 – 15 + 6
= -3
∆₁ = \(\left|\begin{array}{lll}
1 & 1 & 1 \\
6 & 2 & 3 \\
3 & 4 & 9
\end{array}\right|\)
= 1(18 – 12) – 1(54 – 9) + 1(24 – 6)
= 6 – 45 + 18
= -21
∆₂ = \(\left|\begin{array}{lll}
1 & 1 & 1 \\
2 & 6 & 3 \\
1 & 3 & 9
\end{array}\right|\)
= 1(54 – 9) – 1(18 – 3) + 1(6 – 6)
= 45 – 15
= 30
∆₃ = \(\left|\begin{array}{lll}
1 & 1 & 1 \\
2 & 2 & 6 \\
1 & 4 & 3
\end{array}\right|\)
= 1(6 – 24) – 1(6 – 6) + 1(8 – 2)
= -18 – 0 + 6
= -12
x = \(\frac{\Delta_1}{\Delta}=\frac{-21}{-3}\) = 7
y = \(\frac{\Delta_2}{\Delta}=\frac{30}{-3}\) = -10
z = \(\frac{\Delta_3}{\Delta}=\frac{-12}{-3}\) = 4
Solution is x = 7, y = -10, z = 4

Question 22.
Find the shortest distance between the skew lines \(\overline{\mathrm{r}}=(6 \overline{\mathrm{i}}+2 \overline{\mathrm{j}}+2 \overline{\mathrm{k}})+\mathbf{t}(\overline{\mathrm{i}}-2 \overline{\mathrm{j}}+2 \overline{\mathrm{k}})\) and \(\overline{\mathbf{r}}=(-4 \bar{i}-\bar{k})+s(3 \bar{i}-2 \bar{j}-2 \bar{k})\).
Solution:
The first line passes through point A(6, 2, 2) and is parallel to the vector b = i – 2j + 2k.
Second line passes through the point C(-4, 0, -1) and is parallel to the vector d = 3i – 2j – 2k
Shortest distance = \(\frac{|[\mathrm{AC} \quad \mathrm{b} d]|}{|\mathrm{b} \times \mathrm{d}|}\)
d = PQ = ST |cos θ| = \(\frac{\left(b_1 \times b_2\right) \cdot\left(a_2-a_1\right)}{\left|b_1 \times b_2\right|}\)
b × d = \(\left|\begin{array}{ccc}
i & j & k \\
1 & -2 & 2 \\
3 & -2 & -2
\end{array}\right|\) = 8i + 8j + 4k and |b × d| = 12
∴ The shortest distance between the skew lines
\(\frac{|[\mathrm{ACbd}]|}{|\mathrm{b} \times \mathrm{d}|}=\frac{108}{12}\) = 9
[A C b d] = \(\left|\begin{array}{ccc}
-10 & -2 & -3 \\
1 & -2 & 2 \\
3 & -2 & -2
\end{array}\right|\) = -108

Question 23.
If A + B + C = \(\frac{\pi}{2}\) then prove that cos 2A + cos 2B + cos 2C = 1 + 4 sin A sin B sin C.
Solution:
A + B + C = \(\frac{\pi}{2}\) ………(1)
L H S = cos 2A + cos 2B + cos 2C
= 2 cos\(\left(\frac{2 \mathrm{~A}+2 \mathrm{~B}}{2}\right)\) cos\(\left(\frac{2 \mathrm{~A}-2 \mathrm{~B}}{2}\right)\) + cos 2C
= 2 cos (A + B) . cos (A – B) + cos 2C
= 2 cos (90° – C) cos (A – B) + cos 2C
= 2 sin C cos (A – B) + (1 – 2 sin2C)
= 1 + 2 sin C [cos (A – B) – sin C]
= 1 + 2 sin C [cos (A – B) – sin (90° – \(\overline{A+B}\))]
= 1 + 2 sin C [cos (A – B) – cos (A + B)]
= 1 + 2 sin C [2 sin A sin B]
= 1 + 4 sin A sin B sin C
∴ cos 2A + cos 2B + cos 2C = 1 + 4 sin A sin B sin C

Question 24.
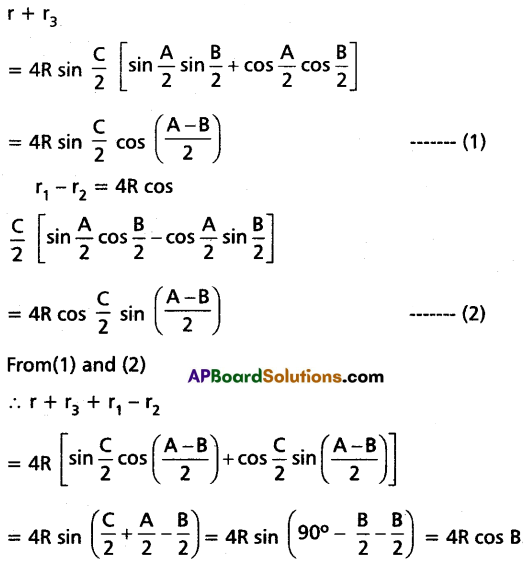
Prove that r + r₃ + r₁ – r₂ = 4R cos B.
Solution: