AP 9th Class Maths Important Questions Chapter 10 Surface Areas and Volumes

These AP 9th Class Maths Important Questions 10th Lesson Surface Areas and Volumes will help students prepare well for the exams.

AP State Syllabus 9th Class Maths 10th Lesson Important Questions and Answers Surface Areas and Volumes

Question 1.
If the surface area of sphere is 616 cm². Find it’s radius.
Solution:
Surface area of sphere = 4πr²
4πr² = 616
4 × \(\frac{22}{7}\) × r² = 616
r² = \(\frac{616 \times 7}{4 \times 22}\) = 49 ⇒ r = 7
∴ Radius = 7 cm.

Question 2.
A sphere and a hemisphere have same radius. What is the ratio of volume of the sphere to volume of the hemisphere?
Solution:
Radius of sphere = r = radius of hemisphere
Volume of sphere = \(\frac{4}{3}\) πr³
Volume of hemisphere = \(\frac{2}{3}\) πr³
∴ Ratios of volumes = \(\frac{4}{3}\) πr³ : \(\frac{2}{3}\) πr³
= 4 : 2 = 2 : 1

Question 3.
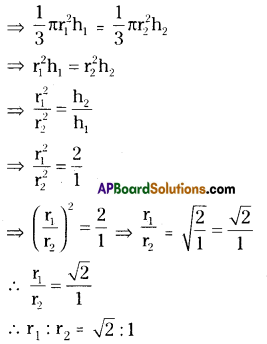
Two right circular cones of equal volumes have their heights in the ratio 1 : 2 then prove that the ratio of their radii is √2 : 1.
Solution:
Let Radii of two right circular cones be r₁ and r₂
Ratio of heights = h₁ and h₂
Ratio of volumes = V₁ and V₂
V₁ = V₂ so,

Question 4.
The surface area of a sphere of radius 5 cm is five times the area of CSA of a cone of radius 5 cm. Then find the volume of the cone.
Solution:
Radius of sphere = r = 5 cm
Surface area of sphere = 4πr²
= 4π(5)² = 100π
Radius of cone = 5 cm
Surface area of cone = πr(r + 1)
= π5(5 + l)
= 5π(5 + l)
Surface area of sphere = 5 x Surface area of cone
100π = 5 x 5π(5 + l)
100π = 25π(5 + l)
5 + l = \(\frac{100 \pi}{25 \pi}\) = 4
l = 4 – 5 = -1 cm
∴ Slant height of a cone is ’-1, so problem solving is not possible.

Question 5.
The volume of a cylinder is 308 cm³. The radius of its base is 3.5 cm. Find its curved surface area and total surface area.
Solution:
Volume of cylinder = πr²h
πr²h = 308
⇒ \(\frac{22}{7}\) × 3.5 × 3.5 × h = 308
⇒ 38.5 × h = 308
⇒ h = \(\frac{308}{38.5}\) = 8cm
CSA of cylinder = 2πrh
= 2 × \(\frac{22}{7}\) × 3.5 × 8
= 2 × 11 × 8 = 196 cm
TSA of cylinder = 2πr (r + h)
= 2 × \(\frac{22}{7}\) × 3.5 (3.5 + 8)
= 2 × 11 (11.5)
= 22 × 11.5 = 253 cm²

Question 6.
Sai, Raju, Latha and Sravani are studying 9th class. In SA-I, Sai got 60 marks out of 80. The average marks of all four students is 48. If the average marks of Sai and Raju is 54 and the average marks of Sai, Raju and Latha is 42 then find the marks obtained by four students individually.
Solution:
Let marks of Sai, Raju, Latha and Sravani be a, b, c, d.
\(\frac{a+b+c+d}{4}\) = 48
a + b + c + d = 192 …………… (1)
= \(\frac{a+b}{2}\) 54 ⇒ a + b = 108 …………….. (2)
\(\frac{a+b+c}{3}\) = 42 ⇒ a + b + c = 126 ……………… (3)
From (2) and (3)
(a + b + c) – (a + b) = 126 – 108 = 18
c = 18
From (1) and (2)
(a + b + c + d) – (a + b + c) = 192 – 126
d = 66
Given that a = 60°
∴ Sai = 60 Marks
Raju = 48 Marks
Latha = 18 Marks
Sravani = 66 Marks