AP State Syllabus SSC 10th Class Maths Solutions 2nd Lesson Sets InText Questions
AP State Board Syllabus AP SSC 10th Class Maths Textbook Solutions Chapter 2 Sets InText Questions and Answers.
10th Class Maths 2nd Lesson Sets InText Questions and Answers
Question 1.
List the teeth under each of the following type (Page No. 25)
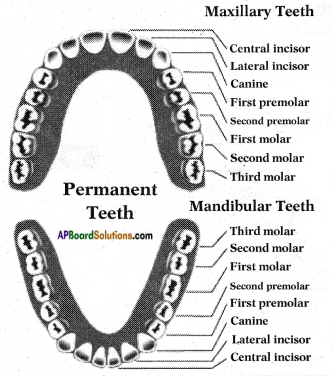
i) Incisors
Answer:
Central incisors = 4
Lateral incisors = 4
Total incisors = 8
ii) Canines
Answer:
Total canines = 4
iii) Pre-molars
Answer:
First premolars = 4
Second premolars = 4
Total premolars = 8
iv) Molars
Answer:
First molars = 4
Second molars = 4
Third molars = 4
Total molars = 12
![]()
Question 2.
Identify and write the “common property” of the following collections. (Page No. 26)
1) 2, 4, 6, 8, …….
Answer:
All are even numbers {x : x is even}
2) 2, 3, 5, 7, 11, …….
Answer:
All are prime numbers (x : x is a prime}
3) 1, 4, 9, 16, …….
Answer:
All are perfect squares
(x : x is a perfect square}
4) January, February, March, April,…
Answer:
All are English months
{x : x is a month of the year}
5) Thumb, index finger, middle finger, ring finger, pinky.
Answer:
All are fingers of a hand
{x : x is a finger of a hand}
Question 3.
Write the following sets. (Page No. 27)
1) Set of the first five positive integers.
Answer:
{11, 2, 3, 4, 5}
2) Set of multiples of 5 which are more than 100 and less than 125.
Answer:
{105, 110, 115, 120}
3) Set of first five cubic numbers.
Answer:
{13, 23, 33, 43, 53}
{1, 8, 27, 64, 125}
4) Set of digits in the Ramanujan number.
Answer:
Ramanujan’s number is 1729
{1, 2, 7, 9}
![]()
Question 4.
Some numbers are given below. Decide the numbers to which number sets they belong to and does not belong to and express with correct symbols. (Page No. 28)
i) 1
Answer:
1 ∈ N
ii) 0
Answer:
0 ∈ W and 0 ∉ N
iii) -4
Answer:
– 4 ∈ I and – 4 ∉ N
iv) \(\frac{5}{6}\)
Answer:
\(\frac{5}{6}\) ∈ Q and \(\frac{5}{6}\) ∉ Z
v) \(1 . \overline{3}\)
Answer:
\(1 . \overline{3}\) ∉ N and \(1 . \overline{3}\) ∉ Z
vi) √2
Answer:
√2 ∈ S and √2 ∉ Q
vii) log 2
Answer:
log 2 ∉ N
viii) 0.03
Answer:
0.03 ∉ Q
ix) π
Answer:
π ∉ Z
x) \(\sqrt{-4}\)
Answer:
\(\sqrt{-4}\) ∉ Q and \(\sqrt{-4}\) ∈ C
Question 5.
List the elements of the following sets. (Page No. 29)
i) G = {all the factors of 20}
ii) F = {the multiples of 4 between 17 and 61 which are divisible by 7}
iii) S = {x : x is a letter in the word ‘MADAM’}
iv) P = {x : x is a whole number between 3.5 and 6.7}
Answer:
i) G = {1, 2, 4, 5, 10, 20}
ii) Multiples of 4 between 17 and 61
x = {20, 24, 28, 32, 36, 40, 44, 48, 52, 56, 60}
F = {28, 56}
iii) S = {M, D, A}
iv) P = {4, 5, 6}
![]()
Question 6.
Write the following sets in the roster form. (Page No. 29)
i) B is the set of all months in a year having 30 days.
ii) P is the set of all prime numbers smaller than 10.
iii) X is the set of the colours of the rainbow.
Answer:
i) B = {April, June, September, November}
ii) P = {2, 3, 5, 7}
iii) X = {Violet, Indigo, Blue, Green, Yellow, Orange, Red}
Question 7.
A is the set of factors of 12. Which one of the following is not a member of A? (Page No. 29)
A) 1
B) 4
C) 5
D) 12
Answer:
[C]
Think & Discuss
Question 1.
Observe the following collections and prepare as many as generalized statements you can describing their more properties. (Page No. 26)
i) 2, 4, 6, 8,….
Answer:
a) All even natural numbers
b) All positive even integers
c) Multiples of 2
ii) 1, 4, 9, 16, …..
Answer:
a) Squares of natural numbers
b) All perfect square numbers
Question 2.
Can you write set of rational numbers listing elements in it? (Page No. 28)
Answer:
We can’t list all elements in the set of rational numbers. We know that rational numbers are infinite.
![]()
Try this
Question 1.
Write some sets of your choice, involving algebraic and geometrical ideas. (Page No. 29)
Answer:
The set of all natural numbers ‘x’ such that 4x + 9 < 50,
ii) A = {x : x is an integer and -3 ≤ x ≤ 7}
iii) B = {Equilateral triangle, Right angled triangle, Scalene triangle, Obtuse angled triangle, Acute angled triangle)
iv) C = {Rectangle, Square, Parallelogram, Rhombus, Trapezium}
Question 2.
Match roster forms with the set builder form. (Page No. 29)
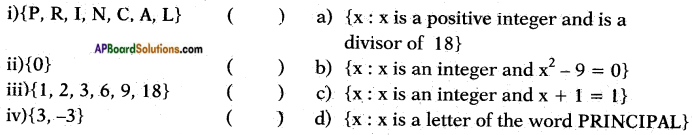
Answer:
i) d
ii) c
iii) a
iv) b
Do this
Question 1.
A = {1, 2, 3, 4},
B = {2, 4},
C = {1, 2, 3, 4, 7}, ∅ = { }.
Fill in the blanks with ⊂ or ⊄. (Page No. 33)
i) A …. B
ii) C …. A
iii) B …. A
iv)A …. C
v) B …. C
vi) ∅ …. B
Answer:
i) A ⊄ B
ii) C ⊄ A
iii) B ⊆ A
iv) A ⊆ C
v) B ⊆ C
vi) ∅ ⊆ B
![]()
Question 2.
State which of the following statements are true. (Page No. 33)
i) { } = ∅
ii) ∅ = 0
iii) 0 = { 0 }
Answer:
i) True (T)
ii) False (F)
iii) False (F)
Question 3.
Let A = {1, 3, 7, 8} and B = [2, 4, 7, 9}.
Find A ∩ B. (Page No. 37)
Answer:
Given sets A = (1, 3, 7, 8} and B = {2,4, 7,9}
A ∩ B = {1, 3, 7, 8} ∩ (2, 4, 7, 9} = {7}
Question 4.
If A = {6,9,11 }; ∅ = {}, find A ∪ ∅, A ∩ ∅). (Page No. 37)
Answer:
Given sets
A = {6, 9, 11} and ∅ = { }
A ∪ ∅ = {6, 9, 11} ∪ { }
= {6, 9, 11} = A
∴ A ∪ ∅ = A
A ∩ ∅ = {6,9,11} ∩ { } = { } = ∅
∴ A ∩ ∅ = ∅
Question 5.
A = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10};
B = {2, 3, 5, 7}. Find A ∩ B and show that A ∩ B = B. (Page No. 37)
Answer:
Given sets
A = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10} and B = {2, 3, 5, 7}
A ∩ B = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10} ∩ {2, 3, 5, 7}
= {2, 3, 5, 7} = B
∴ A ∩ B = B
![]()
Question 6.
If A = {4, 5, 6}; B = {7, 8}, then show that A ∪ B = B ∪ A. (Page No. 37)
Answer:
Given sets are
A = {4, 5, 6} and B = {7, 8}
A ∪ B = {4, 5, 6} ∪ {7, 8}
= {4, 5, 6, 7, 8}.
B ∪ A = {7, 8} ∪ {4, 5, 6}
= {4, 5, 6, 7, 8}
∴ A ∪ B = B ∪ A.
Question 7.
If A = {1, 2, 3, 4, 5 }; B = {4, 5, 6, 7}, then find A – B and B – A. Are they equal? (Page No. 38)
Answer:
Given sets are
A = {1, 2, 3, 4, 5} and B = {4, 5, 6, 7}
A – B = {1, 2, 3, 4, 5} – {4, 5, 6, 7}
= {1, 2, 3}
B – A = {4, 5, 6, 7} – {1, 2, 3, 4, 5}
= {6, 7}
∴ No, A – B ≠ B – A.
Question 8.
If V = {a, e, i, o, u} and B = {a, i, k, u}, find V – B and B – V. (Page No. 38)
Answer:
Given sets are
V = {a, e, i, o, u} and B = {a, i, k, u}
V – B = {a, e, i, o, u} – {a, i, k, u}
= {e, o}
B – V = {a, i, k, u} – {a, e, i, o, u}
= {k}.
Try this
Question 1.
A = {set of quadrilaterals},
B = {square, rectangle, trapezium, rhombus}.
State whether A ⊂ B or B ⊂ A. Justify your answer. (Page No. 33)
Answer:
A = {set of quadrilaterals} means A = {square, rectangle/trapezium, rhombus, parallelogram}
B = {square, rectangle, trapezium, rhombus}
So, B ⊂ A because A’ is having elements more than ‘B’.
![]()
Question 2.
If A = {a, b, c, d}. How many subsets does the set A have? (Page No. 33)
A) 5 B) 6 C) 16 D) 65
Answer:
Given A = {a, b, c, d}
n(A) = 4
Number of subsets for a set, which is having ‘n’ elements is 2n.
So n(A) = 4
Number of subsets for A is 24 = 16.
Answer is [C].
Question 3.
P is the set of factors of 5, Q is the set of factors of 25 and R is the set of factors of 125. Which one of the following is false? (Page No. 33)
A) P ⊂ Q
B) Q ⊂ R
C) R ⊂ P
D) P ⊂ R
Answer: [C]
Question 4.
A is the set of prime numbers smaller than 10, B is the set of odd numbers < 10 and C is the set of even numbers < 10. How many of the following statements are true? (Page No. 33)
i) A ⊂ B
ii) B ⊂ A
iii) A ⊂ C
iv) C ⊂ A
v) B ⊂ C
vi) C ⊂ B
Answer:
All the statements are false.
Question 5.
List out some sets A and B and choose their elements such that A and B are disjoint. (Page No. 37)
Answer:
Consider the disjoint sets
A = {1, 2, 3, 4} and B = {a, b, c}
Question 6.
If A = {2, 3, 5}, find A ∪ ∅ and ∅ ∪ A and compare. (Page No. 37)
Answer:
Given sets A = {2, 3, 5} and ∅ = { }
A ∪ ∅ = {2,3,5} ∪ { } = {2,3,5}
∅ ∪ A = { } ∪ {2, 3, 5} = {2,3,5}
A ∪ v = ∅ ∪ A = A
Question 7.
If A = {1, 2, 3, 4}; B = {1, 2, 3, 4, 5, 6, 7, 8}, then find A ∪ B, A ∩ B. What do you notice about the result? (Page No. 37)
Answer:
Given sets are
A = {1, 2, 3, 4} and B = {1, 2, 3, 4, 5, 6, 7, 8}
A ∪ B = {1, 2, 3, 4} ∪ {1, 2, 3, 4, 5, 6, 7, 8} = {1, 2, 3, 4, 5, 6, 7, 8} = B
A ∩ B = {1, 2, 3, 4} ∩ {1, 2, 3, 4, 5, 6, 7, 8} = {1, 2, 3, 4} = A
If A ⊂ B, then A ∪ B = B and A ∩ B = A
![]()
Question 8.
A = {1, 2, 3, 4, 5, 6}; B = {2, 4, 6, 8, 10}. Find the intersection of A and B. (Page No. 37)
Answer:
Given sets are
A = {1, 2, 3, 4, 5, 6} and B = {2, 4, 6, 8, 10}
A ∩ B = {1, 2, 3, 4, 5, 6} ∩ {2, 4, 6, 8, 10} = {2, 4, 6}
Think & Discuss
Question 1.
Is empty set subset to every set? (Page No. 34)
Answer:
‘Yes’. Empty set is subset to every set
Justify: If A ⊂ B, it means all the elements of set ‘A’ belong to set ‘B’.
In other words we can say no element of ‘A’ missed in set B.
now empty set means which has no elements, now no element of empty set can be missed in any set. So we can write empty set is subset of every set.
Question 2.
Is any set subset to itself? (Page No. 34)
Answer:
‘Yes’. Every set is subset to itself.
Let ‘A’ is any set.
Now every element of ‘A’ definitely belongs to ‘A’.
So A ⊂ A
Hence every set is a subset to it.
Question 3.
You are given two sets such that a set is not a subset of the other. If you have to prove this, how do you prove? (Page No. 34)
Answer:
Let the given sets are ‘A’ and ‘B’.
To prove are set (A) is not subset of other (B).
We check if all elements of ‘A’ belong to the set ‘B’ or not.
If any of the element doesn’t belong to ‘B’ then we can say ‘A’ is not subset of ‘B’. So we have to prove at least one element of ‘A’ does not belong to ‘B’.
Hence ‘A’ is not subset of ‘B’.
Question 4.
The intersection of any two disjoint sets is a null set. Justify your answer. (Page No. 37)
Answer:
Let A and B be any two disjoint sets,
i.e., A and B have no elements in common.
∴ A ∩ B is a null set. (∵ A ∩ B is the set of all elements which are common to both A and B)
![]()
Question 5.
The sets A – B, B – A and A ∩ B are mutually disjoint sets. Use examples to observe if this is true. (Page No. 38)
Answer:
Let the sets are A = {1, 2, 3, 4} and B = {5, 6, 7, 8}
A – B = {1, 2, 3, 4} – {5, 6, 7, 8} = {1, 2, 3, 4}
B – A = {5, 6, 7, 8} – {1, 2, 3, 4} = {5, 6, 7, 8}
A ∩ B = {1, 2, 3, 4} ∩ {5, 6, 7, 8} = { } = ∅
∴ A – B, B – A and A ∩ B are disjoint sets.
Do these
Question 1.
Which of the following are empty sets? Justify your answer. (Page No. 44)
i) Set of integers which lie between 2 and 3 .
ii) Set of natural numbers that are smaller than 1.
iii) Set of odd numbers that leave remainder zero, when divided by 2.
Answer:
i) This is null set. We know that there is no integer that lie between 2 and 3.
ii) This is also a null set. We know that there is’ no natural number less than ‘1’.
iii) This is a null set. We know that odd numbers do not leave remainder zero when divided by 2.
Question 2.
State which of the following sets are finite and which are infinite. Give reasons for your answer. (Page No. 44)
i) A = {x : x e N and x < 100}
ii) B = {x : x e N and x ≤ 5}
iii) C = {12 , 22, 32, ……}
iv) D = {1, 2, 3, 4}
v) {x : x is a day of the week}
Answer:
i) A = (1, 2, 3, 4, , 98, 99}
This set is finite, because there are 99 numbers possible to count.
ii) B = {1, 2, 3, 4, 5}
This set is finite because there are 5 numbers possible to count.
iii) C = {12 , 22, 32, ……}
This set is infinite because there are infinite numbers.
iv) D – {1, 2, 3, 4}
This set is finite because there are 4 numbers that are possible to count.
v) E = {Sunday, Monday, Tuesday, Wednesday, Thursday, Friday, Saturday}
This set is finite, because there are 7 days in a week possible to count.
![]()
Question 3.
Tick the set which is infinite. (Page No. 44)
A) The set of whole numbers < 10
B) The set of prime numbers < 10
C) The set of integers < 10
D) The set of factors of 10
Answer:
[C]
The set of integers < 10
{….., -2, -1, 0, 1, 2, 3, 4, 5, 6, 7, 8, 9}
Try this
Question 1.
Which of the following sets are empty sets? Justify your answer. (Page No. 44)
i) A = {x : x2 = 4 and 3x = 9}.
ii) The set of all triangles in a plane having the sum of their three angles less than 180.
Answer:
i) x2 = 4 ⇒ x = ± 2
3x = 9 ⇒ x = 3
The value of ‘x’ is not same in both cases, so this is a null set.
ii) This is a null set, because the sum of the three angles of a triangle is equal to 180°.
Question 2.
B = {x : x + 5 = 5} is not an empty set. Why? (Page No. 44)
Answer:
B = {x: x + 5 = 5} is not an empty set
let x ∈ Z or x ∈ W
then for x = 0 ⇒ x + 5 = 0 + 5 = 5
So if x ∈ W, or x ∈ Z then for x = 0,
x + 5 = 5 is true.
Then the set B = {0} which is not an empty set.
Note: But if x ∈ N
We will have no ‘x’ such that x + 5 = 5
then ‘B’ will be an empty set.
But in the textbook it is not given whether x ∈ N (or) x ∈ W (or) x ∈ Z.
Hence we consider first one.
![]()
Think & Discuss
Question 1.
An empty set is a finite set. Is this statement true or false? Why? (Page No. 44)
Answer:
Yes, it is a finite set because there is finite number i.e., ‘0’ elements it consists.
Think & Discuss
Question 1.
What is the relation between n(A), n(B), n(A ∩ B) and n(A ∪ B)? (Page No. 45)
Answer:
n(A ∪ B) = n(A) + n(B) – n(A ∩ B). This is called Fundamental theorem of sets.
Question 2.
If A and B are disjoint sets, then how can you find n(A ∪ B)? (Page No. 45)
Answer:
If A and B are disjoint then A ∩ B is a null set.
∴ n(A ∩ B) = 0 and it gives us n(A ∪ B) = n (A) + n(B).
