AP Board 8th Class Maths Solutions Chapter 4 Exponents and Powers Ex 4.1

AP State Syllabus AP Board 8th Class Maths Solutions Chapter 4 Exponents and Powers Ex 4.1 Textbook Questions and Answers.

AP State Syllabus 8th Class Maths Solutions 4th Lesson Exponents and Powers Exercise 4.1

Question 1.
Simplify and give reasons
(i) 4^-3
(ii) (-2) ⁷
(iii) \(\left(\frac{3}{4}\right)^{-3}\)
(iv) (-3)^-4
Solution:
(i) 4^-3 \(\frac{1}{4^{3}}=\frac{1}{64}\) [∵ a^-n = \(\frac{1}{\mathrm{a}^{\prime \prime}}\)

(ii) (-2) ⁷ = -(2) ⁷ = -128
[∵ 7 is an odd number]
[∵ (-a)ⁿ = -(aⁿ) if ‘n’ is odd]

(iii) \(\left(\frac{3}{4}\right)^{-3}\) = \(\frac{3^{-3}}{4^{-3}}=\frac{4^{3}}{3^{3}}=\left(\frac{4}{3}\right)^{3}\)

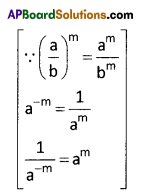
(iv) (-3)^-4 = \(\frac{1}{(-3)^{4}}\) [∵a^-n = \(\frac{1}{a^{n}}\)
= \(\frac{1}{(3)^{4}}\) [∵ 4 is even ]
= \(\frac{1}{81}\)

Question 2.
Simplify the following:
(i) \(\left(\frac{1}{2}\right)^{4} \times\left(\frac{1}{2}\right)^{5} \times\left(\frac{1}{2}\right)^{6}\)
(ii) (-2)⁷ x (-2)³ x (-2)⁴
(iii) 4⁴ x \(\left(\frac{5}{4}\right)^{4}\)
(iv) \(\left(\frac{5^{-4}}{5^{-6}}\right)\) x 5³
(v) (-3) ⁴ x 7⁴
Solution:
(i) \(\left(\frac{1}{2}\right)^{4} \times\left(\frac{1}{2}\right)^{5} \times\left(\frac{1}{2}\right)^{6}\)
\(\left(\frac{1}{2}\right)^{4+5+6}=\left(\frac{1}{2}\right)^{15}=\frac{1}{2^{15}}\)
[∵ a^m x aⁿ = a^{m + n}]

(ii) (-2)⁷ x (-2)³ x (-2)⁴
(-2)^{7 + 3 + 4} = (-2) ¹⁴ = 2 ¹⁴
[∵ (-a)ⁿ = aⁿ is even]

(iii) 4⁴ x \(\left(\frac{5}{4}\right)^{4}\)
4⁴ x \(\left(\frac{5}{4}\right)^{4}\) = 5⁴
[ ∵ \(\left(\frac{a}{b}\right)^{m}=\frac{a^{m}}{b^{m}}\) ]

(iv) \(\left(\frac{5^{-4}}{5^{-6}}\right)\) x 5³
5^-4 x (5⁶ x 5³) [ ∵ \(\frac{1}{a^{-n}}=a^{n}\)
= 5^-4 x 5⁶⁺³ [ ∵ a^m x aⁿ = (a)^m+n
= 5^-4 x 5⁹
= 5^(-4)+9 = 5⁵

(v) (-3) ⁴ x 7⁴
= 3⁴ x 7⁴[.4isevennumber]
=(3 x 7)⁴ [:amxbm=(ab)m]
= (21)⁴

Question 3.
Simplify
(i) \(2^{2} \times \frac{3^{2}}{2^{-2}} \times 3^{-1}\)
(ii) (4^-1 x 3^-1) ÷ 6^-1
Solution:
(i) \(2^{2} \times \frac{3^{2}}{2^{-2}} \times 3^{-1}\)
= 2² x 2² x 3² x 3^-1
= 2²⁺² x 3^{2 + ( – 1)}
=2⁴ x 3¹ = 16 x 3 = 48

(ii) (4^-1 x 3^-1) ÷ 6^-1

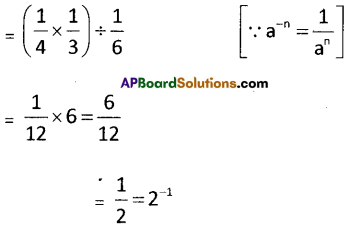
Question 4.
Simplify and give reasons
(i) (4⁰ + 5^-1) x 5² x \(\frac{1}{3}\)
Solution:

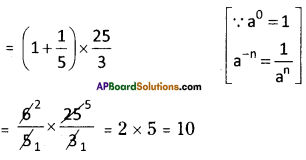
(ii) \(\left(\frac{1}{2}\right)^{-3} \times\left(\frac{1}{4}\right)^{-3} \times\left(\frac{1}{5}\right)^{-3}\)
Solution:
= \(\left(\frac{1}{2} \times \frac{1}{4} \times \frac{1}{5}\right)^{-3}\)
= \(\left(\frac{1}{40}\right)^{-3}\) [∵ a^m x b^m x c^m = (abc)^m
= (40)³ [ ∵]\(\frac{1}{a^{-n}}\) = aⁿ ]

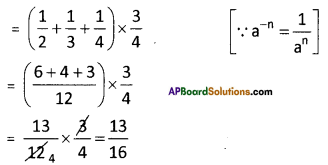
(iii) (2^-1 + 3^-1 + 4^-1) x \(\frac{3}{4}\)
Solution:

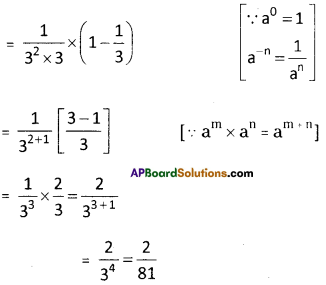
(iv) \(\frac{3^{-2}}{3}\) x (3⁰ – 3^-1
Solution:

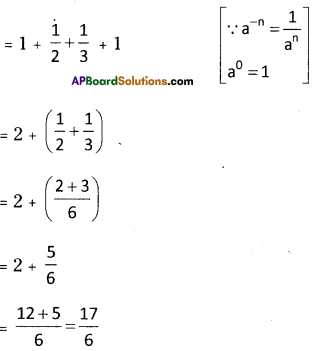
(v) 1 + 2^-1 + 3^-1 + 4⁰
Solution:

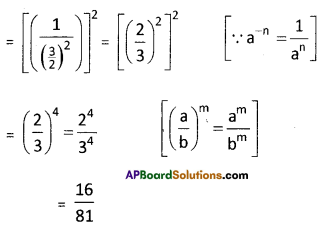
(vi) \(\left[\left(\frac{3}{2}\right)^{-2}\right]^{2}\)
Solution:

Question 5.
Simplify and give reasons
(i) \(\left[\left(3^{2}-2^{2}\right) \div \frac{1}{5}\right]^{2}\)
Solution:
\(\left.\left[(9-4) \div \frac{1}{5}\right)\right]^{2}\)
= \(\left[5 \times \frac{5}{1}\right]^{2}\) = (5²)² 5⁴ = 625 [∵ (a^m)ⁿ = a^mn]

(ii) ((5²)³ x 5⁴) ÷ 5⁶
Solution:

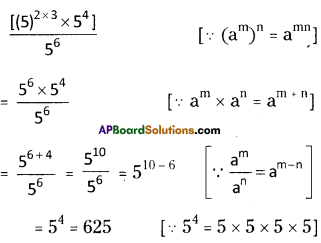
Question 6.
Find the value of ’n’ in each of the following:
(i) \(\left(\frac{2}{3}\right)^{3} \times\left(\frac{2}{3}\right)^{5}=\left(\frac{2}{3}\right)^{\mathrm{n}-2}\)
Solution:

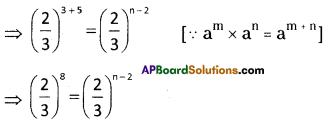
Here bases are equal, so exponents are
also equal.
⇒ n – 2 = 8
⇒ n = 8 + 2 = 10
∴ n = 10

(ii) (-3)ⁿ⁺¹ x (-3)⁵ = (-3)³
Solution:
⇒(-3)ⁿ⁺¹⁺⁵ = (-3)^-4 [∵ a^m x aⁿ = a^m+n ]
⇒ (-3)ⁿ⁺⁶ = (-3)^-4
⇒ n + 6 = -4
⇒ n = -4 – 6 = -10
⇒ n = -10

(iii) 7²ⁿ⁺¹ ÷ 49 = 7³
Solution:

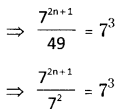
⇒ 7^2n+1-2 = 7³ [ ∵ \(\frac{a^{m}}{a^{n}}=a^{m-n}\) ]
⇒ 7^{2n – 1}= 7³
⇒ 2n – 1 = 3
⇒ 2n = 3 + 1 = 4
⇒ n = \(\frac{4}{2}\)
∴ n = 2

Question 7.
Find ’x’ if 2^-3 = \(\frac{1}{2^{x}}\)
Solution:
2^-3 = \(\frac{1}{2^{x}}\) = 2^-x
⇒ 2^-3 = 2^-x [ \(\frac{1}{a^{n}}\) = a^-n ]
⇒ -x = -3
∴ x = 3

Question 8.
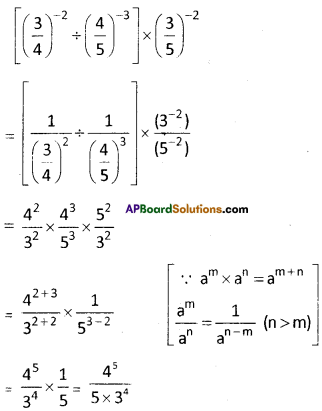
Simplify \(\left[\left(\frac{3}{4}\right)^{-2} \div\left(\frac{4}{5}\right)^{-3}\right] \times\left(\frac{3}{5}\right)^{-2}\)
Solution:

Question 9.
If m = 3 and n = 2 find the value of
(i) 9m² – 10n³
(ii) 2m² n²
(iii) 2m³ + 3n² – 5m²n
(iv) mⁿ – n^m
Solution:
1) 9m² – 10n³
= 9(3)² – 10(2)³
= 9 x 9 – 10 x8
= 81 – 80 = 1

(ii) 2m² n²
= 2(3)² (2)²
= 2 x 9 x 4 = 72

(iii) 2m³ + 3n² – 5m²n
= 2(3)³ + 3(2)² – 5(3)²(2)
= (2 x 27) + (3 x 4) – (5 x 9 x 2)
= 54 + 12 – 90
= 66 – 90 = – 24

(iv) mⁿ – n^m
= 3² – 2³
= 3 x 3 – 2 x 2 x 2
= 9 – 8 = 1

Question 10.
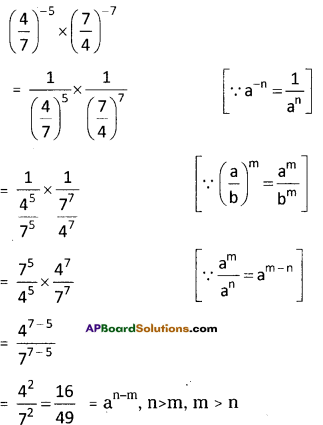
Simplify and give reasons \(\left(\frac{4}{7}\right)^{-5} \times\left(\frac{7}{4}\right)^{-7}\)
Solution: