AP 9th Class Maths Important Questions Chapter 3 The Elements of Geometry

These AP 9th Class Maths Important Questions 3rd Lesson The Elements of Geometry will help students prepare well for the exams.

AP State Syllabus 9th Class Maths 3rd Lesson Important Questions and Answers The Elements of Geometry

Question 1.
Write converse of the theorem “In ΔABC, if AB = AC then C = ∠B”.
Solution:
In ΔABC, ∠C = ∠B then AB = AC.

Question 2.
Write converse of the theorem “In ΔABC, if AB = AC then ∠C = ∠B”.
Solution:
In ΔABC, ∠C = ∠B then AB = AC.

Question 3.
Write any two Euclid’s postulates.
Solution:
i) There is a unique line that passes through the given two distinct points.
ii) We can draw a circle with any centre and radius.

Question 4.
Write any two Euclid’s axioms.
Solution:
i) Things which are equal to the same things are equal to one another.
ii) Things which coincide with one another are equal to one another.
iii) The whole is greater than the part.

Question 5.
If x + a is a common factor of f(x) = x² + x – 6 and g(x) = x² + 3x – 18, then find the value of a.
Solution:
given polynomials are f(x) = x² + x – 6 and g(x) = x² + 3x – 18
f(x) and g(x) having common factors (x + a), So
x + a = 0 ⇒ x = -a
f(-a) = (-a)²+ (-a) – 6
= a² – a – 6
g(-a) = (-a)² + 3(-a) – 18
= a² – 3a – 18
f(-a) = g(-a)
a² – a – 6 = a² – 3a – 18
a² – a² – a + 3a = -18 + 6
2a = -12
a = -6.

Question 6.
If a + b = 5 and a² + b² = 11,then prove that a³ + b³ = 20
Solution:
Given a + b = 5 and
a² + b² = 11
(a + b)² = (5)²
a² + b² + 2ab = 25
11 + 2ab = 25
2ab = 14
ab = 7
a³ + b³ = (a + b) (a² + b² – ab)
= 5(11 – 7)
= 5 × 4 = 20
∴ a³ + b³ = 20.

Question 7.
Disprove that “a² > b² for all a > b” by . finding a suitable counter example.
Solution:
If a > b so,
Let a = 3 and b = 2
a² = 3² and b² = 2²
9 > 4 so,
∴ If a > b, then a² > b².

Question 8.
Explain Euclid’s 5^thpostulate with the help of a diagram.
Solution:
Euclid’s 5th postulate : If a straight line , falling on two straight lines makes the interior angles on the same side of it taken together is less than two right angles, then the two straight lines, if produced infinitely, meet on that side on \frhich the sum of the angles is less than two right angles.

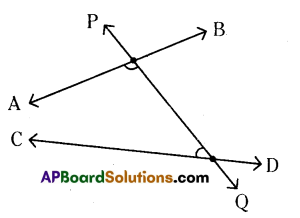
The lines AB and CD will intersect on left side of PQ.